Advertisements
Advertisements
प्रश्न
A machine is set to deliver packets of a given weight. Ten samples of size five each were recorded. Below are given relevant data:
| Sample number | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| `bar"X"` | 15 | 17 | 15 | 18 | 17 | 14 | 18 | 15 | 1 | 16 |
| R | 7 | 7 | 4 | 9 | 8 | 7 | 12 | 4 | 11 | 5 |
Calculate the control limits for mean chart and the range chart and then comment on the state of control, (conversion factors for n = 5, A2 = 0.58, D3 = 0 and D4 = 2.115)
उत्तर
| Sample number | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | Tota |
| Mean `(bar"X")` | 15 | 17 | 15 | 18 | 17 | 14 | 18 | 15 | 1 | 16 | `sum"X"` = 162 |
| Range (R) | 7 | 7 | 4 | 9 | 8 | 7 | 12 | 4 | 11 | 5 | `sum"R"` = 74 |
The control limits for `bar"X"` chart is
`\overset{==}{"X"} = (sum"X")/"No. of samples" = 162/10` = 16.2
`bar"R" = (sum"R")/"No. of samples" = 74/10` = 7.4
UCL = `\overset{==}{"X"} + "A"_2+ bar"R"`
= 16.2 + (0.58)(7.4)
= 16.2 + 4.292
= 20.492
= 20.49
CL = `\overset{=}{"X"}` = 16.2
LCL = `\overset{==}{"X"} - "A"_2 bar"R"`
= 16.2 – (0.58)(7.4)
= 16.2 – 4.292
= 11.908
= 11.91
The control limits for Range chart is
CL = `"D"_4bar"R"`
= (2.115)(7.4)
= 15.651
= 15.65
CL = `bar"R"` = 7.4
LCL = `"D"_3 bar"R"` = (0)(7.4) = 0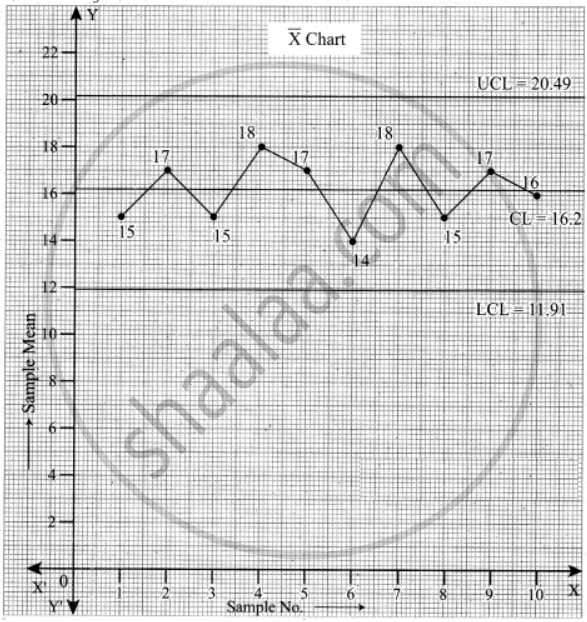
The above diagram shows all the three control lines with the data points plotted.
We see that all the points of the sample mean are within the control limits.
We now draw the R chart for the given data.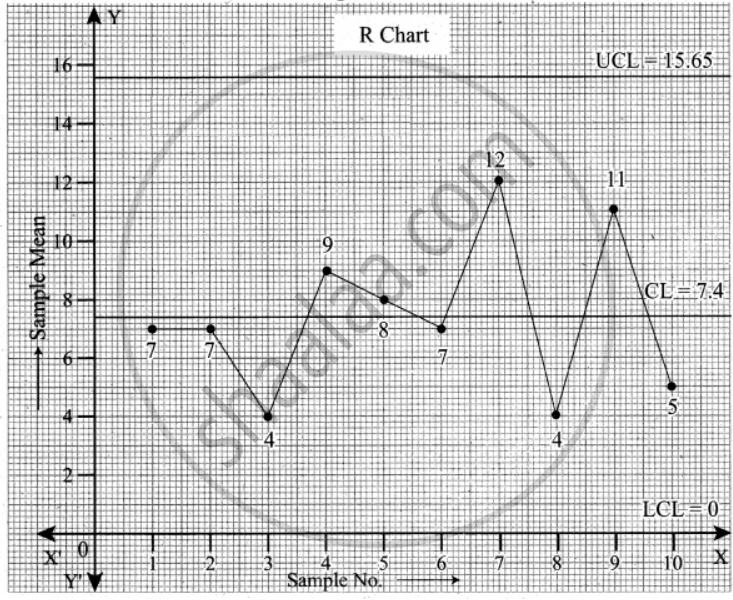
The above diagram shows all the three control lines with the sample range points plotted.
We observe that all the points are within the control limits.
Conclusion: From the above two plots of the sample mean `bar"X"` and sample range R, we conclude that the process is in control.
APPEARS IN
संबंधित प्रश्न
Mention the types of causes for variation in a production process
Define assignable cause
What do you mean by product control?
What do you mean by process control?
Name the control charts for variables
Define the mean chart
Define R chart
The following data show the values of sample means and the ranges for ten samples of size 4 each. Construct the control chart for mean and range chart and determine whether the process is in control.
| Sample Number | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| `bar"X"` | 29 | 26 | 37 | 34 | 14 | 45 | 39 | 20 | 34 | 23 |
| R | 39 | 10 | 39 | 17 | 12 | 20 | 05 | 21 | 23 | 15 |
Choose the correct alternative:
The assignable causes can occur due to
Choose the correct alternative:
The LCL for R chart is given by
