Question

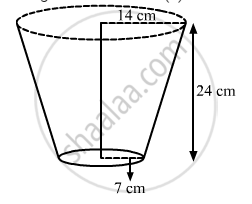
A metallic bucket, open at the top, of height 24 cm is in the form of the frustum of a cone, the radii of whose lower and upper circular ends are 7 cm and 14 cm respectively. Find :
(i) the volume of water which can completely fill the bucket.
(ii) the area of the metal sheet used to make the bucket.
[Use π =\[\frac{22}{7}\]

Answer 1

Upper radius of the bucket (R) = 14 cm
Lower radius of the bucket (r) = 7 cm
Height of the bucket (h) = 24 cm

Slant height of the bucket (l) =\[\sqrt{h^2 + (R - r )^2}\]

= \[\sqrt{{24}^2 + (14 - 7 )^2} cm\]

=\[\sqrt{576 + 49} cm\]

= \[\sqrt{625} cm\]

= 25 cm

(i) We know that the volume of a bucket is given by:

\[V = \frac{1}{3}\pi\left( R^2 + Rr + r^2 \right)h\]

\[\Rightarrow V = \left\{ \frac{1}{3}\pi\left( {14}^2 + 14 \times 7 + 7^2 \right) \right\}24 {cm}^3 \]

\[ = \left( \frac{1}{3} \times \frac{22}{7} \times 343 \times 24 \right) {cm}^3 \]

\[ = 8624 {cm}^3\]

Thus, the volume of water that can completely fill the bucket is 8624 cm³.

(ii) To measure the area of the metal sheet used in making the bucket, we need to find the surface area of the bucket.
Surface area of the bucket = Curved surface area of the frustum + Curved surface area of the circular base

=\[\pi\left( R + r \right)l + \pi r^2\]

=\[\left( \frac{22}{7} \times \left( 14 + 7 \right) \times 25 + \frac{22}{7} \times 7^2 \right) {cm}^2\]

= \[\left( 1650 + 154 \right) {cm}^2\]

=\[1804 {cm}^2\]

Thus, the area of the metallic sheet used to make the bucket is 1804 cm².