Advertisements
Advertisements
प्रश्न
A non-uniform bar of weight W is suspended at rest by two strings of negligible weight as shown in the figure. The angles made by the strings with the vertical are 36.9° and 53.1° respectively. The bar is 2 m long. Calculate the distance d of the centre of gravity of the bar from its left end.
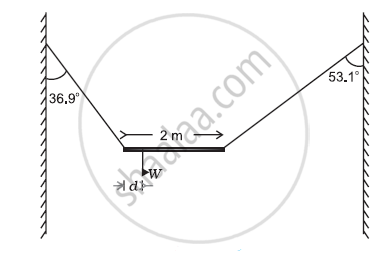
उत्तर
The free body diagram of the bar is shown in the following figure.
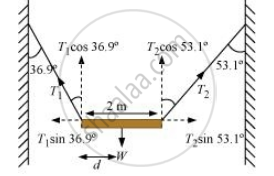
Length of the bar, l = 2 m
T1 and T2 are the tensions produced in the left and right strings respectively.
At translational equilibrium, we have:
`T_1 sin 36.9^@ = T_2 sin 53.1`
`T_1/T_2 = (sin 53.1^@)/(sin 36.9)`
`= (0.800)/0.600 = 4/3`
`=> T_1 = 4/3 T_2`
For rotational equilibrium, on taking the torque about the centre of gravity, we have:
`T_1 cos 36.9 xx d = T_2 cos 53.1 (2-d)`
`T_1 xx 0.800d = T_2 0.600(2-d)`
`4/3xxT_2xx0.800d = T_2[0.600xx2- 0.600d]`
`1.067 d + 0.6d = 1.2`
`∴ d = 1.2/1.67`
= 0.72 m
Therefore, the center of gravity (C.G.) of the specified bar is located 0.72 meters from its left end.
APPEARS IN
संबंधित प्रश्न
A sphere is rotating about a diameter.
Two particles of equal masses have velocity `vecv_1 = 2hati` m/s and `vecv_2 = 2hatj` m/s. The first particle has an acceleration `veca_1 = (3hati + 3hatj)` m/s2 while the acceleration of the other particle is zero. The centre of mass of the two particles moves in a
Radium nucleus was moving with constant velocity v. It disintegrates into He and Rn. He and Rn move as shown in the figure. The CM of the system of He and Rn will move along ...A ... after the explosion of Ra. Here, A refers to:

