Advertisements
Advertisements
प्रश्न
A rectangular loop of size l × b carrying a steady current I is placed in a uniform magnetic field `vecB`. Prove that the torque `vectau`acting on the loop is give by `vectau =vecm xx vecB,`where `vecm` is the magnetic moment of the loop.
Advertisements
उत्तर
Plane of the loop is at an angle with the direction of the magnetic field,
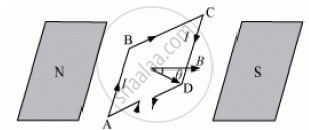
Let the angle between the field and the normal is θ. The forces on BC and DA are equal and opposite and they cancel each other as they are collinear.
Force on AB is F1 and force on CD is F2, thus
F1 = F2 = IbB
Magnitude of torque on the loop as in the figure:
`because tau =F_1 1/2 sintheta +F_2 1/2 sintheta =IlBsintheta`
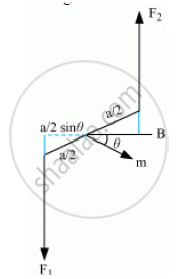
or, τ = IAB sin θ (Where area,A = lb)
If there are ‘n’ such turns the torque will be nIAB sinθ
Magnetic moment of the current, m = IA
`vectau=vecm xx vecB`
संबंधित प्रश्न
The rectangular wire-frame, shown in figure, has a width d, mass m, resistance R and a large length. A uniform magnetic field B exists to the left of the frame. A constant force F starts pushing the frame into the magnetic field at t = 0. (a) Find the acceleration of the frame when its speed has increased to v. (b) Show that after some time the frame will move with a constant velocity till the whole frame enters into the magnetic field. Find this velocity v0. (c) Show that the velocity at time t is given by
v = v0(1 − e−Ft/mv0).
Figure shows a square loop of edge a made of a uniform wire. A current i enters the loop at the point A and leaves it at the point C. Find the magnetic field at the point P which is on the perpendicular bisector of AB at a distance a/4 from it.
Find the magnetic field B at the centre of a rectangular loop of length l and width b, carrying a current i.
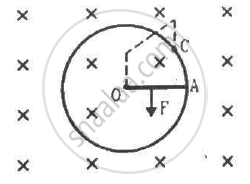
Figure shows a conducting circular loop of radius a placed in a uniform, perpendicular magnetic field B. A thick metal rod OA is pivoted at the centre O. The other end of the rod touches the loop at A. The centre O and a fixed point C on the loop are connected by a wire OC of resistance R. A force is applied at the middle point of the rod OAperpendicularly, so that the rod rotates clockwise at a uniform angular velocity ω. Find the force.
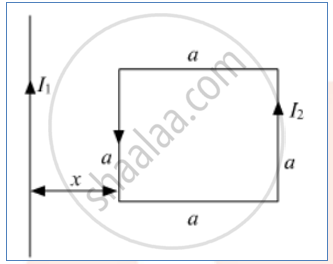
A square loop of side 'a' carrying a current I2 is kept at distance x from an infinitely long straight wire carrying a current I1 as shown in the figure. Obtain the expression for the resultant force acting on the loop.
A planar loop of rectangular shape is moved within the region of a uniform magnetic field acting perpendicular to its plane. What is the direction and magnitude of the current induced in it?
A rectangular conducting loop consists of two wires on two opposite sides of length l joined together by rods of length d. The wires are each of the same material but with cross-sections differing by a factor of 2. The thicker wire has a resistance R and the rods are of low resistance, which in turn are connected to a constant voltage source V0. The loop is placed in uniform a magnetic field B at 45° to its plane. Find τ, the torque exerted by the magnetic field on the loop about an axis through the centres of rods.
A vector quantity that measures the strength and orientation of a current loop as a magnetic source is called the:
The rotational effect experienced by a current-carrying loop placed in a uniform magnetic field is called:
In a rectangular loop ABCD placed in a uniform magnetic field \[\vec{B}\], the sides AB and CD are parallel to \[\vec{B}\]. The force on each of these sides is:
