Advertisements
Advertisements
प्रश्न
A steel wire of mass µ per unit length with a circular cross section has a radius of 0.1 cm. The wire is of length 10 m when measured lying horizontal, and hangs from a hook on the wall. A mass of 25 kg is hung from the free end of the wire. Assuming the wire to be uniform and lateral strains << longitudinal strains, find the extension in the length of the wire. The density of steel is 7860 kg m–3 (Young’s modules Y = 2 × 1011 Nm–2).
उत्तर
Consider the diagram when a small element of length dx is considered at x from the load (x = 0).

Let T(x) and T(x + dx) are tensions on the two cross-sections a distance dx apart, then – t(x + dx) + T(x) = dmg = μ dxg (where μ is the mass/length) ......(∵ dm = μdx)
dT = μgdx .....[∵ dT = T(x + dx) – T(x)]
⇒ T(x) = μgx + C .....(On integrating)
At x = 0, T(0) = Mg
⇒ C = mg
∴ T(x) = μgx + Mg
Let the length dx at x increase by dr, then
Young's modulus Y = `"Stress"/"Strain"`
`((T(x))/A)/((dr)/(dx)) = Y`
⇒ `(dr)/(dx) = 1/(YA) T(x)`
⇒ `r = 1/(YA) int_0^L (μgx + Mg)dx`
= `1/(YA) [(μgx^2)/2 + Mgx]_0^L`
= `1/(YA)[(mgL^2)/2 + MgL]` ......(m is the mass of the wire)
`A = pi xx (10^-3)^2 m^2`
`Y = 200 xx 10^9 Nm^-2`
`m = pi xx (10^-3)^2 xx 10 xx 7860` kg
∴ `r = 1/(2 xx 10^11 xx pi xx 10^-6)` ......`[(pi xx 786 xx 10^-3 xx 10 xx 10)/2 + 25 xx 10 xx 10]`
= `[196.5 xx 10^-6 + 3.98 xx 10^-3]`
= 4 × 10–3 m
APPEARS IN
संबंधित प्रश्न
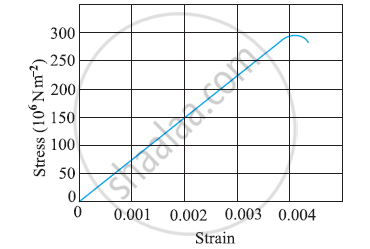
The figure shows the strain-stress curve for a given material. What are (a) Young’s modulus and (b) approximate yield strength for this material?
The stress-strain graphs for materials A and B are shown in Figure
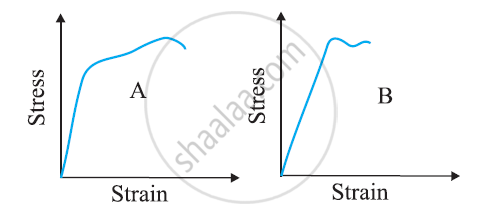
The graphs are drawn to the same scale.
(a) Which of the materials has the greater Young’s modulus?
(b) Which of the two is the stronger material?
Read the following statements below carefully and state, with reasons, if it is true or false
The Young’s modulus of rubber is greater than that of steel;
Young's modulus of a perfectly rigid body is ______.
Identical springs of steel and copper are equally stretched. On which, more work will have to be done?
What is the Young’s modulus for a perfect rigid body ?
A truck is pulling a car out of a ditch by means of a steel cable that is 9.1 m long and has a radius of 5 mm. When the car just begins to move, the tension in the cable is 800 N. How much has the cable stretched? (Young’s modulus for steel is 2 × 1011 Nm–2.)
If the yield strength of steel is 2.5 × 108 Nm–2, what is the maximum weight that can be hung at the lower end of the wire?
If the length of a wire is made double and the radius is halved of its respective values. Then, Young's modules of the material of the wire will ______.
The force required to stretch a wire of cross section 1 cm2 to double its length will be ______.
(Given Young's modulus of the wire = 2 × 1011 N/m2)
