Advertisements
Advertisements
प्रश्न
ABC is a right triangle such that AB = AC and bisector of angle C intersects the side AB at D. Prove that AC + AD = BC.
Advertisements
उत्तर
Given: In right angled ΔABC, AB = AC and CD is the bisector of ∠C.
Construction: Draw DE ⊥ BC.
To prove: AC + AD = BC
Proof: In right angled ΔABC, AB = AC and BC is a hypotensue ...[Given]
∴ ∠A = 90°
In ΔDAC and ΔDEC, ∠A = ∠3 = 90°
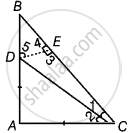
∠1 = ∠2 ...[Given, CD is the bisector of ∠C]
DC = DC ...[Common sides]
∴ ΔDAC ≅ ΔDEC ...[By AAS congruence rule]
⇒ DA = DE [By CPCT] ...(i)
And AC = EC ...(ii)
In ΔABC, AB = AC
∠C = ∠B [Angles opposite to equal sides are equal] ...(iii)
Again, in ΔABC, ∠A + ∠B + ∠C = 180° ...[By angles sum property of a triangle]
⇒ 90° + ∠B + ∠B = 180° ...[From equation (iii)]
⇒ 2∠B = 180° – 90°
⇒ 2∠B = 90°
⇒ ∠B = 45°
In ΔBED, ∠5 = 180° – (∠B + ∠4) ...[By angle sum property of a triangle]
= 180° – (45° + 90°)
= 180° – 135°
= 45°
∴ ∠B = ∠5
⇒ DE = BE [∵ Sides opposite to equal angles are equal] ...(iv)
From equations (i) and (iv),
DA = DE = BE ...(v)
∵ BC = CE + EB
= CA + DA ...[From equations (ii) and (v)]
∴ AD + AC = BC
Hence proved.
APPEARS IN
संबंधित प्रश्न
ABC is a right angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
ΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see the given figure). If AD is extended to intersect BC at P, show that
- ΔABD ≅ ΔACD
- ΔABP ≅ ΔACP
- AP bisects ∠A as well as ∠D.
- AP is the perpendicular bisector of BC.
Two sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of ΔPQR (see the given figure). Show that:
- ΔABM ≅ ΔPQN
- ΔABC ≅ ΔPQR
BE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles.
ABC is an isosceles triangle with AB = AC. Drawn AP ⊥ BC to show that ∠B = ∠C.
ABC is an isosceles triangle in which AC = BC. AD and BE are respectively two altitudes to sides BC and AC. Prove that AE = BD.
Prove that sum of any two sides of a triangle is greater than twice the median with respect to the third side.
In a right triangle, prove that the line-segment joining the mid-point of the hypotenuse to the opposite vertex is half the hypotenuse.
Two lines l and m intersect at the point O and P is a point on a line n passing through the point O such that P is equidistant from l and m. Prove that n is the bisector of the angle formed by l and m.
Line segment joining the mid-points M and N of parallel sides AB and DC, respectively of a trapezium ABCD is perpendicular to both the sides AB and DC. Prove that AD = BC.
