Advertisements
Advertisements
प्रश्न
Arrive at an expression for elastic collision in one dimension and discuss various cases.
उत्तर
Consider two elastic bodies of masses m1 and m2 moving in a straight line (along positive x-direction) on a frictionless horizontal surface as shown in the figure given below.
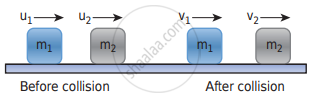
Elastic collision in one dimension
| Mass | Initial velocity | Final velocity |
| Mass m1 | u1 | v1 |
| Mass m2 | u2 | v2 |
In order to have a collision, we assume that the mass m] moves faster than mass m2 i.e., u1 > u2. For elastic collision, the total linear momentum and kinetic energies of the two bodies before and after a collision must remain the same.
| The momentum of mass m1 | Momentum of mass m2 | Total linear momentum | |
| Before collision | Pi1 = m1u1 | Pi2 = m2u2 | `P_i = P_{i1} + P_{i2}` |
| `P_i = m_1u_1 + m_2u_2` | |||
| After Collision | Pf1 = m1v1 | Pf2 = m2v2 | `P_f = P_{f1} + P_{f2}` |
| `P_f = m_1v_1 + m_2v_2` |
From the law of conservation of linear momentum,
Total momentum before collision (pi) = Total momentum after collision (pf)
m1u1 + m2u2 = m1v1 + m2v2 …(i)
Or `m_1(u_1 - v_1) = m_2(v_2 - u_2)` ..................(ii)
Further,
| Kinetic energy of mass `m_1` | Kinetic energy of mass `m_2` | Total kinetic energy | |
| Before collision | `KE_{i1} = 1/2m_1u_1^2` | `KE_{i2} = 1/2m_2u_2^2` |
`KE_i = KE_{i1} + KE_{i2}` `KE_i = 1/2m_1u_1^2 + 1/2m_2u_2^2` |
| After collision | `KE_{f1} = 1/2m_1v_1^2` | `KE_{f2} = 1/2m_2v_2^2` |
`KE_i = KE_{i1} + KE_{i2}` `KE_f = 1/2m_1v_1^2 + 1/2m_2v_2^2` |
For elastic collision,
Total kinetic energy before collision `KE_i` = Total kinetic energy after collision `KE_f`
`1/2m_1u_1^2 + 1/2m_2u_2^2 = 1/2m_1v_1^2 + 1/2m_2v_2^2` .....................(iii)
After simplifying and rearranging the terms,
`m_1(u_1^2 - v_1^2) = m_2(v_2^2 - u_2^2)`
Using the formula `a^2 - b^2` = (a + b)(a - b), we can rewrite the above equation as
`m_1(u_1 + v_1)(u_1 - v_1) = m_2(v_2 + u_2)(v_2 - u_2)` .......................(iv)
Diving equation (iv) by (ii) gives,
`(m_1(u_1 + v_1)(u_1 - v_1))/(m_1(u_1 - v_1)) = (m_2(v_2 + u_2)(v_2 - u_2))/(m_2(v_2 - u_2))`
`u_1 + v_1 = v_2 + u_2`
`u_1 - u_2 = v_2 - v_1` .............Rearranging, (v)
Equation (v) can be rewritten as
`u_1 - u_2 = -(v_1 - v_2)`
This means that for any elastic head-on collision, the relative speed of the two elastic bodies after the collision has the same magnitude as before collision but in opposite direction. Further, note that this result is independent of mass.
Rewriting the above equation for v1 and v2,
v1 = v2 + u2 – u2 …(vi)
Or v2 = u1 + v1 – u2 …(vii)
To find the final velocities v1 and v2:
Substituting equation (vii) in equation (ii) gives the velocity of as m1 as
m1 (u1 – v1) = m2(u1 + v1 – u2 – u2)
m1 (u1 – y1) = m2 (u1 + + v1 – 2u2)
m1u1 – m1v1 = m2u1 + m2v1 + 2m2u2
m1u1 – m2u1 + 2m2u2 = m1v1 + m2v1
(m1– m2) u1 + 2m2u2 = (m1 + m2) v1
Or `v_1 = ((m_1 - m_2)/(m_1 + m_2))u_1 + ((2m_2)/(m_1 + m_2))u_2` ................(viii)
Similarly, by substituting (vi) in equation (ii) or substituting equation (viii) in equation (vii), we get the final velocity of m2 as
`v_2 = ((2m_1)/(m_1 + m_2))u_1 + ((m_2 - m_1)/(m_1 + m_2))u_2` ....................(ix)
Case 1: When bodies has the same mass i.e., m1 = m2,
equation (viii) ⇒ `v_1 = (0)u_1 + ((2m_2)/(2m_2))u_2`
`v_1 = u_2` .............................(x)
equation (ix) ⇒ `v_2 = ((2m_1)/(2m_1))u_1 + (0)u_2`
`v_2 = u_1` .............................(xi)
The equations (x) and (xi) show that in a one-dimensional elastic collision when two bodies of equal mass collide after the collision their velocities are exchanged.
Case 2: When bodies have the same mass i.e., m1 = m2 and second body (usually called target) is at rest (u2 = 0),
By substituting m1 = m2 = and u2 = 0 in equations (viii) and equations (ix) we get,
from equation (viii) ⇒ v1 = 0 …(xii)
from equation (ix) ⇒ v2 = u1 ….. (xiii)
Equations (xii) and (xiii) show that when the first body comes to rest the second body moves with the initial velocity of the first body.
Case 3: The first body is very much lighter than the second body
(m1 << m2, `m_1/m_2` << 1) then the ratio `m_1/m_2 ≈ 0` and also if the target is at rest (`u_2 = 0`)
Dividing numerator and denominator of equation (viii) by m2, we get
`v_1 = ((m_1/m_2 - 1)/(m_1/m_2 + 1))u_1 + (2/(m_1/m_2 + 1))`(0)
`v_1 = ((0 - 1)/(0 + 1))u_1`
`v_1 = -u_1`
Similarly the numerator and denominator of equation (ix) by m2, we get
`v_2 = ((2m_1/m_2)/(m_1/m_2 + 1))u_1 + ((1 - m_1/m_2)/(m_1/m_2 + 1))`(0)
`v_2 = (0)u_1 + ((1 - m_1/m_2)/(m_1/m_2 + 1))`(0)
`v_2 = 0` ................................(xv)
The equation (xiv) implies that the first body which is lighter returns back (rebounds) in the opposite direction with the same initial velocity as it has a negative sign. The equation (xv) implies that the second body which is heavier in mass continues to remain at rest even after the collision. For example, if a ball is thrown at a fixed wall, the ball will bounce back from the wall with the same velocity with which it was thrown but in opposite direction.
Case 4: The second body is very much lighter than the first body (m2 << m1, `m_2/m_1` << 1) then the ratio `m_2/m_1 = 0` and also if the target is at rest `u_2 = 0`
Dividing numerator and denominator of equation (viii) by `m_1`, we get
`v_1 = ((1 - m_2/m_1)/(1 + m_2/m_1))u_1 + ((2m_2/m_1)/(1 + m_2/m_1))`(0)
`v_1 = ((1 - 0)/(1 + 0))u_1 + (0/(1 + 0))`(0)
`v_1 = u_1` .............(xvi)
Similarly,
Dividing numerator and denominator of equation (xiii) by m1, we get
`v_2 = (2/(1 + m_2/m_1))u_1 + ((m_2/m_1 - 1)/(1 + m_2/m_1))`(0)
`v_2 = (2/(1 + 0))u_1`
`v_2 = 2u_1` ...........(xvii)
The equation (xvi) implies that the first body which is heavier continues to move with the same initial velocity. The equation (xvii) suggests that the second body which is lighter will move with twice the initial velocity of the first body. It means that the lighter body is thrown away from the point of collision.
APPEARS IN
संबंधित प्रश्न
State if the following statement is true or false. Give a reason for your answer.
Total energy of a system is always conserved, no matter what internal and external forces on the body are present.
State if the following statement is true or false. Give a reason for your answer.
In an inelastic collision, the final kinetic energy is always less than the initial kinetic energy of the system.
Which of the following potential energy curves in Fig. cannot possibly describe the elastic collision of two billiard balls? Here r is distance between centres of the balls.

Consider the decay of a free neutron at rest : n → p + e–
Show that the two-body decay of this type must necessarily give an electron of fixed energy and, therefore, cannot account for the observed continuous energy distribution in the β-decay of a neutron or a nucleus

Two different unknown masses A and B collide. A is initially at rest when B has a speed v. After collision B has a speed v/2 and moves at right angles to its original direction of motion. Find the direction in which A moves after the collision.
A ball is thrown vertically down from height of 80 m from the ground with an initial velocity 'v'. The ball hits the ground, loses `1/6`th of its total mechanical energy, and rebounds back to the same height. If the acceleration due to gravity is 10 ms-2, the value of 'v' is
A particle of mass 'm' collides with another stationary particle of mass 'M'. A particle of mass 'm' stops just after collision. The coefficient of restitution is ______.
Two pendulums with identical bobs and lengths are suspended from a common support such that in rest position the two bobs are in contact (Figure). One of the bobs is released after being displaced by 10° so that it collides elastically head-on with the other bob.

- Describe the motion of two bobs.
- Draw a graph showing variation in energy of either pendulum with time, for 0 ≤ t ≤ 2T, where T is the period of each pendulum.
A particle of mass m with an initial velocity u`hat"i"` collides perfectly elastically with a mass 3m at rest. It moves with a velocity v`hat"j"` after collision, then, v is given by :
A sphere of mass 'm' moving with velocity 'v' collides head-on another sphere of same mass which is at rest. The ratio of final velocity of second sphere to the initial velocity of the first sphere is ______. ( e is coefficient of restitution and collision is inelastic)
