Advertisements
Advertisements
प्रश्न
Considering the case of a parallel plate capacitor being charged, show how one is required to generalize Ampere's circuital law to include the term due to displacement current.
उत्तर
Using Gauss’ law, the electric flux ΦE of a parallel plate capacitor having an area A, and a total charge Q is
`phi_E=EA=1/in_0Q/AxxA`
`=Q/in_0`
Where electric field is
`E=Q/(Ain_0)`
As the charge Q on the capacitor plates changes with time, so current is given by
i = dQ/dt
`:.(dphi_E)/dt=d/dt(Q/in_0)=1/in_0(dQ)/dt`
`=>in_0(dphi_E)/dt=(dQ)/dt=i`
This is the missing term in Ampere’s circuital law.
So the total current through the conductor is
i = Conduction current (ic) + Displacement current (id)
`:.i=i_c+i_d=i_c+in_0(dphi_E)/dt`
As Ampere’s circuital law is given by
`:.ointvecB.vec(dl)=mu_0I`
After modification we have Ampere−Maxwell law is given as
`ointB.dl=mu_0i_c+mu_0in_0(dphi_E)/dt`
The total current passing through any surface, of which the closed loop is the perimeter, is the sum of the conduction and displacement current.
APPEARS IN
संबंधित प्रश्न
Draw a neat labelled diagram of a parallel plate capacitor completely filled with dielectric.
Explain briefly the process of charging a parallel plate capacitor when it is connected across a d.c. battery
What is the area of the plates of a 2 F parallel plate capacitor, given that the separation between the plates is 0.5 cm? [You will realize from your answer why ordinary capacitors are in the range of µF or less. However, electrolytic capacitors do have a much larger capacitance (0.1 F) because of very minute separation between the conductors.]
Show that the force on each plate of a parallel plate capacitor has a magnitude equal to `(1/2)` QE, where Q is the charge on the capacitor, and E is the magnitude of the electric field between the plates. Explain the origin of the factor `1/2`.
A parallel plate capacitor is to be designed with a voltage rating 1 kV, using a material of dielectric constant 3 and dielectric strength about 107 Vm−1. (Dielectric strength is the maximum electric field a material can tolerate without breakdown, i.e., without starting to conduct electricity through partial ionisation.) For safety, we should like the field never to exceed, say 10% of the dielectric strength. What minimum area of the plates is required to have a capacitance of 50 pF?
A slab of material of dielectric constant K has the same area as the plates of a parallel plate capacitor but has a thickness \[\frac{3d}{4}\]. Find the ratio of the capacitance with dielectric inside it to its capacitance without the dielectric.
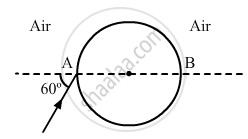
A ray of light falls on a transparent sphere with centre C as shown in the figure. The ray emerges from the sphere parallel to the line AB. Find the angle of refraction at A if the refractive index of the material of the sphere is \[\sqrt{3}\].
A parallel-plate capacitor is charged to a potential difference V by a dc source. The capacitor is then disconnected from the source. If the distance between the plates is doubled, state with reason how the following change:
(i) electric field between the plates
(ii) capacitance, and
(iii) energy stored in the capacitor
Answer the following question.
Describe briefly the process of transferring the charge between the two plates of a parallel plate capacitor when connected to a battery. Derive an expression for the energy stored in a capacitor.
Solve the following question.
A parallel plate capacitor is charged by a battery to a potential difference V. It is disconnected from the battery and then connected to another uncharged capacitor of the same capacitance. Calculate the ratio of the energy stored in the combination to the initial energy on the single capacitor.
