Advertisements
Advertisements
प्रश्न
Derive an expression for the maximum line of sight distance between two antennas for space wave propagation.
उत्तर
Maximum line of sight distance:
The transmitting and receiving antennae are shown below.

Consider only the transmitter antenna. From the geometry of the figure, we get
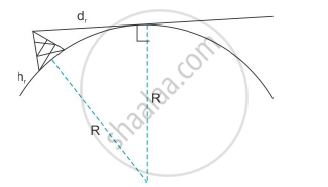
`(R+h_r)^2=R^2+d_r^2`
`:.R^2+2h_rR +h_r^2=R^2 +d_r^2`
But, we know that `h_r^2 "<< "2h_rR`
∴ `R^2+2h_rR=R^2+d_r^2`
`:.d_r^2=2h_rR`
`:.d_r=sqrt(2h_rR)`
This is the distance between the transmitter and horizon.
Similarly, for the receiver, we have `d_r=sqrt(2h_rR)`
Hence, the maximum line-of-sight distance between the transmitter and the receiver is
`d=d_r+d_R=sqrt(2Rh_r)+sqrt(2Rh_R)`
APPEARS IN
संबंधित प्रश्न
Write a short note on surface wave propagation of electromagnetic waves.
Line of sight propagation is also called as_________________ .
The outermost layer of the earth’s atmosphere is _______.
(A) stratosphere
(B) mesospher
(C) troposphere
(D) ionosphere
State three components of space wave propagation.
Explain why this mode cannot be used for long distance communication using high frequencies.
An em wave is travelling in a medium with a velocity `vecv =vhati` Draw a sketch showing the propagation of the em wave, indicating the direction of the oscillating electric and magnetic fields.
Mention three different modes of propagation used in communication system.
Polarization in e.m wave is caused by
A TV transmission tower has a height of 240 m. Signals broadcast from this tower will be received by LOS communication at a distance of (assume the radius of earth to be 6.4 × 106 m)
- 100 km
- 24 km
- 55 km
- 50 km
(i) The intensity of a light pulse travelling along a communication channel decreases exponentially with distance x according to the relation I = Ioe–αx, where Io is the intensity at x = 0 and α is the attenuation constant. Show that the intensity reduces by 75 per cent after a distance of `((In 4)/α)`
(ii) Attenuation of a signal can be expressed in decibel (dB) according to the relation dB = `10 log_10 (I/I_0)`. What is the attenuation in dB/km for an optical fibre in which the intensity falls by 50 per cent over a distance of 50 km?
