Question

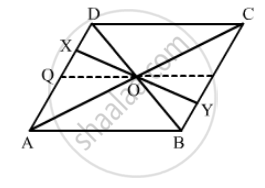
Diagonals of parallelogram ABCD intersect at O as shown in the following fegure. XY contains O, and X, Y are points on opposite sides of the parallelogram. Give reasons for each of the following:
(i) OB = OD
(ii) ∠OBY = ∠ODX
(iii) ∠BOY = ∠DOX
(iv) ∆BOY ≅ ∆DOX
Now, state if XY is bisected at O.

 

Answer 1

(i) Diagonals of a parallelogram bisect each other.
(ii) Alternate angles
(iii) Vertically opposite angles
(iv)\[\text{ In } ∆ BOY \text{ and } ∆ DOX: \]
\[OB = OD (\text{ diagonals of a parallelogram bisect each other })\]
\[\angle OBY = \angle ODX (alternate angles)\]
\[\angle BOY = \angle DOX (\text{ vertically opposite angles })\]

ASA congruence:
XO = YO (c.p.c.t)
So, XY is bisected at O.