Question

Draw a pair of vertically opposite angles. Bisect each of the two angles. Verify that the bisecting rays are in the same line.

Answer 1

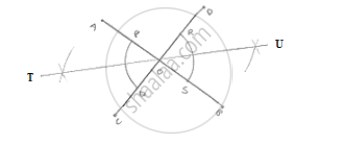
Steps of construction:
1. Draw a pair of vertically opposite angle AOC and DOB
2. With center O and any radius drawn two arcs which intersect OA at P, Q-OB at S and OD at R.
3. With center P and Q and radius more than `1/2`PQ draw two arcs which intersect each other at 7.
4. Join to

5. With center R and S radius more than `1/2`RS,  draw two arcs which intersect each other at U.
6. Join OU. 
  ∴ TOU is a straight line