Advertisements
Advertisements
प्रश्न
Draw the diagram of a single movable pulley and obtain its mechanical advantage, velocity ratio, and efficiency.
उत्तर
Since it is inconvenient to apply the effort in an upward direction, a single fixed pulley is used to change the direction of effort.


In equilibrium, L = T + T = 2T
E = T
Mechanical advantage =`"Load(L)"/"Effort(E)"=(2"T")/"T"=2`
As the effort is pulled down through a distance dE, the two segments of the thread carrying the movable pulley and the load goes up by dE/2.
Hence, dL = dE/2
Velocity ratio (VR) = `"d"_"E"/"d"_"L"`
= `"d"_"E"/("d"_"E"/2)`
= 2.
Efficiency =`"MA"/"VR"`
= `2/2`
= 1 or 100%.
APPEARS IN
संबंधित प्रश्न
Answer the following in short.
Explain the various functions that a machine can perform.
Explain how a gear system can be used to obtain gain in speed? Given one example.
Define the following term in reference to a gear system for Gear ratio ?
In which direction does the force need to applied, when a single pulley is used with a mechanical advantage greater than one? How can you change the direction of force applied without altering its mechanical advantage? Draw a labelled diagram of the system.
Give a reason for the following:
In a single fixed pulley, the velocity ratio is always more than the mechanical advantage.
Name a machine which is used to:
change the direction of force applied.
Draw a diagram of a single fixed pulley and obtain expressions for its:
(i) Mechanical advantage,
(ii) Velocity ratio, and
(iii) Efficiency, in the ideal case.
Diagram given below shows an arrangement of four pulleys. A load L is attached to the movable lower block and effort E is applied at the free end of the string.
Copy the diagram; and
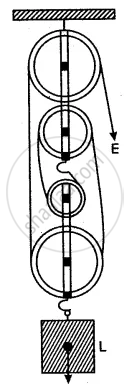
(i) Draw arrows to indicate tension in each part of the string; and
(ii) Calculate the mechanical advantage of the system.
In a single movable pulley system, a load of 125 kgf is lifted by an effort of 75 kgf. Find the percentage efficiency of system.
A ‘block and tackle’ system used 3 pulleys in the lower block and 4 pulleys in the upper block. What is the ‘velocity ratio’ of this system? If the load is to be lifted by a person capable of applying a maximum effort of 1000 N, what is the maximum load than can be lifted under ideal conditions?
The actual maximum load that gets lifted turns out to be 6300 N. What are the values of the actual M.A. and efficiency of the set-up?
