Advertisements
Advertisements
प्रश्न
Find the number of side of a regular polygon, when of its angle has a measure of 162° .
Advertisements
उत्तर
\[ \text{ Each interior angle } = \left( \frac{2n - 4}{n} \times 90 \right)^° \]
\[So, \left( \frac{2n - 4}{n} \times 90 \right)^° = 162° \]
\[ \Rightarrow \frac{2n - 4}{n} = \frac{162° }{90° }\]
\[ \Rightarrow \frac{2n - 4}{n} = \frac{9}{5}\]
\[ \Rightarrow 10n - 20 = 9n\]
\[ \therefore n = 20\]
संबंधित प्रश्न
Examine the table. (Each figure is divided into triangles and the sum of the angles deduced from that.)
| Figure |  |
 |
 |
 |
| Side | 3 | 4 | 5 | 6 |
| Angle sum | 180° |
2 × 180° = (4 − 2) × 180° |
3 × 180° = (5 − 2) × 180° |
4 × 180° = (6 − 2) × 180° |
What can you say about the angle sum of a convex polygon with number of sides?
a) 7
b) 8
c) 10
d) n
Classify the following curve as open or closed:
Classify the following curve as open or closed:
Classify the following curve as open or closed:
Illustrate, if posible, one of the following with a rough diagram:
A colsed curve that is not a polygon.
Find the number of side of a regular polygon, when of its angle has a measure of 135° .
Find the number of degrees in each exterior exterior angle of a regular pentagon.
A nonagon has ______ sides.
ABCDE is a regular pentagon. The bisector of angle A meets the side CD at M. Find ∠AMC.
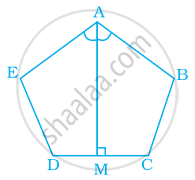
What is the maximum exterior angle possible for a regular polygon?
