Advertisements
Advertisements
प्रश्न
Find one irrational number between 0.2101 and 0.222... = `0.bar2`
उत्तर
Let
a = 0.2101
b = 0.2222... = `0.bar2`
Here a and b are rational numbers .Since a has terminating and b has repeating decimal. We observe that in second decimal place a has 1 and b has 2. So a < b.
Hence one irrational number is 0.220100100010000... lying between 0.2101 and 0.2222...
APPEARS IN
संबंधित प्रश्न
State whether the following statement is true or false. Justify your answer.
Every irrational number is a real number.
Examine, whether the following number are rational or irrational:
`sqrt3+sqrt2`
Identify the following as rational or irrational number. Give the decimal representation of rational number:
`sqrt4`
Show that (5 - 2`sqrt(3)`) is irrational.
Write a pair of irrational numbers whose sum is irrational.
Write the following in descending order:
`5sqrt(3), sqrt(15) and 3sqrt(5)`
Insert five irrational number's between `2sqrt(3) and 3sqrt(5)`.
The product `root(3)(2) * root(4)(2) * root(12)(32)` equals to ______.
Classify the following number as rational or irrational with justification:
`sqrt(12)/sqrt(75)`
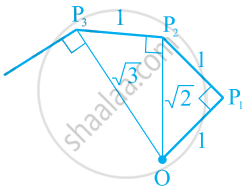
Classroom activity (Constructing the ‘square root spiral’): Take a large sheet of paper and construct the ‘square root spiral’ in the following fashion. Start with a point O and draw a line segment OP1 of unit length. Draw a line segment P1 P2 perpendicular to OP1 of unit length. Now draw a line segment P2 P3 perpendicular to OP2. Then draw a line segment P3 P4 perpendicular to OP3. Continuing in this manner, you can get the line segment Pn–1Pn by drawing a line segment of unit length perpendicular to OPn–1. In this manner, you will have created the points P2, P3,...., Pn,.... ., and joined them to create a beautiful spiral depicting `sqrt2, sqrt3, sqrt4,` ...