Question

Find the expression for the capacitance of a parallel plate capacitor of plate area A and plate separation d when 

1. a dielectric slab of thickness t and 
2. a metallic slab of thickness t,

where (t < d) are introduced one by one between the plates of the capacitor. In which case would the capacitance be more and why?

Answer 1

I. The capacitance of a parallel plate capacitor with dielectric slab (t < d)

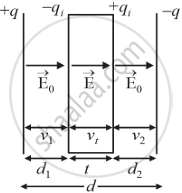
+q, −q = The charges on the capacitor plates

+q_i, −q_i = Induced charges on the faces of the dielectric slab

E₀ → Electric field intensity in air between the plates

E → The reduced value of electric field intensity inside the dielectric slab.

When a dielectric slab of thickness t < d is introduced between the two plates of the capacitor, the electric field reduces to E due to the polarisation of the dielectric. The potential difference between the two plates is given by

V = V₁ + V_t + V₂

V = E₀d₁ + E_t + E₀d₂   ...(1)

Here E is the reduced value of electric field intensity, `vecE = vecE_0 + vecE_i`.

Here `vecE_i` is the electric field due to the induced charges [+q_i and −q_i].

E = `sqrt(E_0^2 + E_i^2 + 2E_0E_i cos180^circ)`

= `sqrt((E_0 - E_i)^2)`

E = E₀ − E_i

Also, the dielectric constant K is given by,

`K = E_0/E`   ...(2)

`E_0 = sigma/epsilon_0 = q/(Aepsilon_0)`   ...(3)

From equations (1), (2) and (3)

`V = E_0[d_1 + d_2] + E_0/Kt`

`V = q/(Aepsilon_0)[d - t + t/K]`   ...(4)

The capacitance of the capacitor on the introduction of the dielectric slab is

`C = q/V`   ...(5)

From (4) and (5)

`C = (epsilon_0A)/(d - t + t/k)`

If t = d, then `C = k(epsilon_0A)/(d)` ⇒ C = KC₀ 

Here `C_0 = (epsilon_0A)/d`

Since K > 1, therefore C > C₀

II. For a metallic slab, K is infinitely large, therefore `C = (epsilon_0A)/(d - t)`.