Advertisements
Advertisements
प्रश्न
For each of the following statements state whether true(T) or false (F)
The length of the line segment joining the midpoints of any two sides of a triangles is equal to half the length of the third side.
उत्तर
True
Suppose ABC is a triangle and M, N are
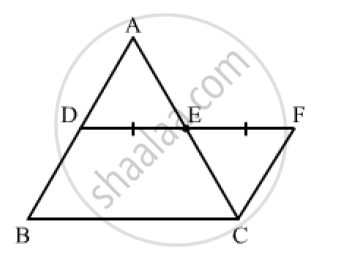
Construction: DE is expanded to F such that EF = DE
To proof = DE =` 1/2`𝐵𝐶
Proof: In ΔADE and ΔCEF
AE = EC (E is the mid point of AC)
DE = EF (By construction)
AED = CEF (Vertically Opposite angle)
By SAS criterion, ΔADE ~= ΔCEF
CF = AD (CPCT)
⟹ BD = CF
∠𝐴𝐷𝐸= ∠𝐸𝐹𝐶 (𝐶𝑃𝐶𝑇)
Since, ∠𝐴𝐷𝐸 𝑎𝑛𝑑 ∠𝐸𝐹𝐶 𝑎𝑟𝑒 𝑎𝑙𝑡𝑒𝑟𝑛𝑎𝑡𝑒 𝑎𝑛𝑔𝑙𝑒
Hence, AD ‖ CF and BD ‖ CF
When two sides of a quadrilateral are parallel, then it is a parallelogram
∴ DF = BC and BD ‖ CF
∴BDFC is a parallelogram
Hence, DF = BC
⟹ DE + EF = BC
⟹ 𝐷𝐸=`1/2` 𝐵𝐶
APPEARS IN
संबंधित प्रश्न
All squares are ______.
All ______ triangles are similar.
State whether the following quadrilaterals are similar or not:

Write the truth value (T/F) of each of the following statement
Two polygons are similar if their corresponding angles are proportional.
For each of the following statements state whether true(T) or false (F)
In a ΔABC , AB = 6 cm, ∠A = 45° and AC = 8 cm and in a ΔDEF , DF = 9 cm ∠D = 45° and DE= 12 cm then ΔABC ∼ΔDEF
For each of the following statements state whether true(T) or false (F)
the polygon formed by joining the midpoints of the sides of a quadrilateral is a rhombus.
For each of the following statements state whether true(T) or false (F)
the ratio of the perimeter of two similar triangles is the same as the ratio of the their corresponding medians.
The symbol ~ is used to represent _________ triangles
If in two As ABC and DEF, `"AB"/"DF" = "BC"/"FE" = "CA"/"ED"`, then ______.
In Figure, ∠BAC = 90° and AD ⊥ BC. Then, ______.