Advertisements
Advertisements
प्रश्न
In Figure, ∠BAC = 90° and AD ⊥ BC. Then, ______.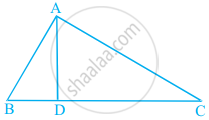
पर्याय
BD . CD = BC2
AB . AC = BC2
BD . CD = AD2
AB . AC = AD2
उत्तर
In Figure, ∠BAC = 90° and AD ⊥ BC. Then, BD . CD = AD2.
Explanation:

From ∆ADB and ∆ADC,
According to the question, we have,
∠D = ∠D = 90° ...(∵ AD ⊥ BC)
∠DBA = ∠DAC ...[Each angle = 90° – ∠C]
Using AAA similarity criteria,
∆ADB ∼ ∆ADC
`("BD")/("AD") = ("AD")/("CD")`
BD . CD = AD2
APPEARS IN
संबंधित प्रश्न
Give two different example of a pair of similar figures.
Two triangles are similar, if their corresponding sides are .......... (proportional, equal)
Write the truth value (T/F) of each of the following statement
Any two congruent figures are similar.
Write the truth value (T/F) of each of the following statement
Two polygons are similar if their corresponding angles are proportional.
Write the truth value (T/F) of each of the following statement
Two triangles are similar if their corresponding angles are proportional.
For each of the following statements state whether true(T) or false (F)
if two triangles are similar then their corresponding angles are equal and their corresponding sides are equal
For each of the following statements state whether true(T) or false (F)
In a ΔABC , AB = 6 cm, ∠A = 45° and AC = 8 cm and in a ΔDEF , DF = 9 cm ∠D = 45° and DE= 12 cm then ΔABC ∼ΔDEF
Similar triangles have the same ________ but not necessarily the same size
If in two As ABC and DEF, `"AB"/"DF" = "BC"/"FE" = "CA"/"ED"`, then ______.
Observe the figures given below carefully and answer the questions:
| Figure A | 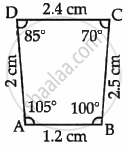 |
 |
| Figure B |  |
 |
| Figure C | 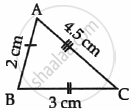 |
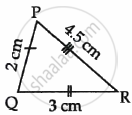 |
- Name the figure(s) where in two figures are similar.
- Name the figure(s) where in the figures are congruent.
-
- Prove that congruent triangles are also similar but not the converse.
OR - What more is least needed for two similar triangles to be congruent?
- Prove that congruent triangles are also similar but not the converse.
