Advertisements
Advertisements
प्रश्न
Four point charges Q, q, Q and q are placed at the corners of a square of side 'a' as shown in the figure.

Find the
1) resultant electric force on a charge Q, and
2) potential energy of this system.
उत्तर

1) `F_"net" = "KQ"^2/(2a^2) + (sqrt2KQq)/a^2`
2) Potential energy of the system = `(4KQq)/a + "KQ"^2/(sqrt2a) + (Kq^2)/(sqrt2a)`
APPEARS IN
संबंधित प्रश्न
If one of the two electrons of a H2 molecule is removed, we get a hydrogen molecular ion `"H"_2^+`. In the ground state of an `"H"_2^+`, the two protons are separated by roughly 1.5 Å, and the electron is roughly 1 Å from each proton. Determine the potential energy of the system. Specify your choice of zero potential energy.
Find out the amount of the work done to separate the charges at infinite distance.
A point charge Q is placed at point O as shown in the figure. The potential difference VA – VB positive. Is the charge Q negative or positive?

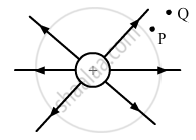
Figure shows the field lines due to a positive point charge. Give the sign of potential energy difference of a small negative charge between the points Q and P.
If a charge q0 is there in an electric field caused by several point charges qi. The potential energy of q0 is given by ________.
Consider a uniform electric field in the z-direction. The potential is a constant ______.
In the circuit shown in figure initially, key K1 is closed and key K2 is open. Then K1 is opened and K2 is closed (order is important). [Take Q1′ and Q2′ as charges on C1 and C2 and V1 and V2 as voltage respectively.]

Then
- charge on C1 gets redistributed such that V1 = V2
- charge on C1 gets redistributed such that Q1′ = Q2′
- charge on C1 gets redistributed such that C1V1 + C2V2 = C1E
- charge on C1 gets redistributed such that Q1′ + Q2′ = Q
Calculate potential energy of a point charge – q placed along the axis due to a charge +Q uniformly distributed along a ring of radius R. Sketch P.E. as a function of axial distance z from the centre of the ring. Looking at graph, can you see what would happen if – q is displaced slightly from the centre of the ring (along the axis)?
- Assertion (A): Work done in moving a charge around a closed path, in an electric field is always zero.
- Reason (R): Electrostatic force is a conservative force.
Charges (+q) and (–q) are placed at points A and B respectively which are a distance 2L apart. C is the midpoint between A and B. What is the work done in moving a charge +Q along the semicircle CRD?