Advertisements
Advertisements
प्रश्न
How does the time period (T) of a simple pendulum depend on its length (l)? Draw a graph showing the variation of T2 with l. How will you use this graph to determine the value of g (acceleration due to gravity)?
उत्तर
The time period of a simple pendulum is directly proportional to the square root of its effective length.
`T ∝ sqrt(l)`
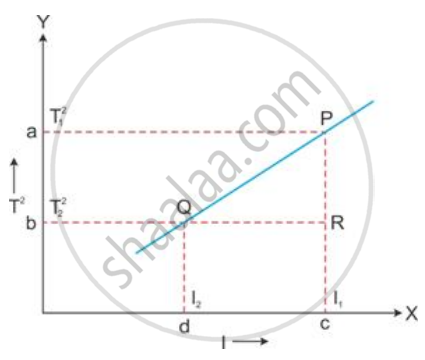
From this graph, the value of acceleration due to gravity (g) can be calculated as follows.
The slope of the straight line can be found by taking two points P and Q on the straight line and drawing normals from these points on the X- and Y-axis, respectively. Then, the value of T2 is to be noted at a and b, the value of l at c and d. Then,
Slope = `"PR"/"QR" = "ab"/"cd" = (T_1^2 - T_2^2)/(l_1 - l_2)`
This slope is found to be constant at a place and is equal to `(4pi^2)/"g"` , where g is the acceleration due to gravity at that place. Thus, g can be determined at a place from these measurements using the following relation:
`"g" = (4pi^2)/("Slope" "of" T^2 "Vs" l "graph")`
APPEARS IN
संबंधित प्रश्न
A microscope is provided with a main scale graduated with 20 divisions in 1 cm and a vernier scale with 50 division on it of length same as of 49 divisions of main scale. Find the least count of the microscope.
State the numerical value of the frequency of oscillation of a second's pendulum. Does it depend on the amplitude of oscillations?
A seconds pendulum is taken to a place where acceleration due to the gravity falls to one-fourth. How is the time period of the pendulum affected, if at all? Give reasons. What will be its new time period?
Name the most convenient unit of mass you will use to measure:
Mass of small amount of medicine.
Name the most convenient unit of mass you will use to measure:
The grain output of a state
Name the most convenient unit of mass you will use to measure:
The bag of sugar
Explain the method in steps to find the volume of an irregular solid with the help of a measuring cylinder.
Which of the following measurement is most accurate?
