Advertisements
Advertisements
प्रश्न
If a, b, c, d are in continued proportion, prove that: `(a^3 + b^3 + c^3)/(b^3 + c^3 + d^3) = a/d`
उत्तर
a, b, c, d are in continued proportion
∴ `a/b = b/c = c/d` = k(say)
∴ c = dk, b = ck = dk, k = dk2
a = bk = dk2. k = dk3
L.H.S. = `(a^3 + b^3 + c^3)/(b^3 + c^3 + d^3)`
= `((dk^3)^3 + (dk^2)^3 + (dk)^3)/((dk^2)^3 + (dk)^3 + d^3)`
= `(d^3k^9 + d^3k^6 + d^3k^3)/(d^3k^6 + d^3k^3 + d^3)`
= `(d^3k^3(k^6 + k^3 + 1))/(d^3(k^6 + k^3 + 1)`
= k3
R.H.S. = `a/d`
= `(dk^3)/d`
= k3
∴ L.H.S. = R.H.S.
APPEARS IN
संबंधित प्रश्न
Find the fourth proportional to 3a, 6a2 and 2ab2
If a, b, c are in continued proportion and a(b - c) = 2b, prove that `a - c = (2(a + b))/a`
Find the third proportion to the following :
`9/25` and `18/25`
Find the mean proportion of the following :
0.09 and 0.25
If a, b, c, d are in continued proportion, prove that:
(a2 + b2 + c2) (b2 + c2 + d2) = (ab + bc + cd)2.
Find the third proportional to Rs. 3, Rs. 12
If the cost of a dozen soaps is Rs 285.60, what will be the cost of 15 such soaps?
Choose the correct answer from the given options :
The third proportional to `6(1)/(4)` and 5 is
Are 30, 40, 45, and 60 in proportion?
Sleeping time of a python in a 24 hour clock is represented by the shaded portion in figure.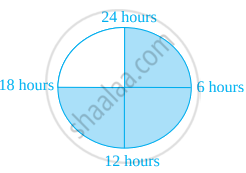
The ratio of sleeping time to awaking time is ______.
