Advertisements
Advertisements
प्रश्न
If the lines kx – y + 4 = 0 and 2y = 6x + 7 are perpendicular to each other, find the value of k.
उत्तर
Given lines are
kx – y + 4 = 0
And 2y = 6x + 7
Or y = kx + 4 ...(i)
And y = `3x + 7/2` ...(ii)
On comparing with y = mx + c, we get
m1 = k and m2 = 3
If lines are perpendicular, then
m1m2 = – 1
`\implies` k × 3 = – 1
`\implies` k = `(-1)/3`
APPEARS IN
संबंधित प्रश्न
Find the slope of the line parallel to AB if : A = (0, −3) and B = (−2, 5)
Without using the distance formula, show that the points A(4, 5), B(1, 2), C(4, 3) and D(7, 6) are the vertices of a parallelogram.
Fill in the blank using correct alternative.
Out of the following, point ........ lies to the right of the origin on X– axis.
Show that A(4, –1), B(6, 0), C(7, –2) and D(5, –3) are vertices of a square.
Find the slope of the diagonals of a quadrilateral with vertices A(1, 7), B(6, 3), C(0, –3) and D(–3, 3).
Find the slope of a line passing through the following pair of point
(²m²,2am) and (p²m²,2pm)
Find the slope and the y-intercept of the following line x - 2 = `(5 - 3"y")/2`
A line passing through the points (a, 2a) and (- 2, 3) is perpendicular to the line 4a + 3y + 5 = 0. Find the value of a.
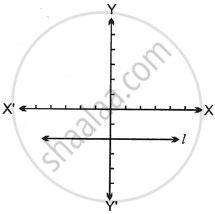
In the figure, line l is parallel to X-axis. Which of the following statement is true?