Advertisements
Advertisements
प्रश्न
In ΔABC, D and E are the mid-point on AB and AC such that DE || BC.
If AD : BD = 4 : 5 and EC = 2.5cm, find AE.
उत्तर
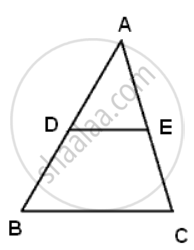
In ΔADE and ΔABC
∠D = ∠B and ∠C = ∠E ...(DE || BC)
⇒ ΔADE ∼ ΔABC
∴ `"AD"/"DB" = "AE"/"EC"`
⇒ `(4)/(5) = "AE"/(2.5)`
⇒ AE = `(4 xx 2.5)/(5)`
⇒ AE = 2cm.
APPEARS IN
संबंधित प्रश्न
In the following figure, A, B and C are points on OP, OQ and OR respectively such that AB || PQ and AC || PR. Show that BC || QR.

Given: FB = FD, AE ⊥ FD and FC ⊥ AD.
Prove that: `(FB)/(AD) = (BC)/(ED)`.

In the given figure, triangle ABC is similar to triangle PQR. AM and PN are altitudes whereas AX and PY are medians.
prove that
`("AM")/("PN")=("AX")/("PY")`
P and Q are points on the sides AB and AC respectively of a ΔABC. If AP = 2cm, PB = 4cm, AQ = 3cm and QC = 6cm, show that BC = 3PQ.
In a right triangle ABC, right angled at B, D is a point on hypotenuse such that BD ⊥ AC , if DP ⊥ AB and DQ ⊥ BC then prove that
`(a) DQ^2 Dp.QC (b) DP ^2 DQ.AP 2 `

In Δ ABC, D and E are points on the sides AB and AC respectively. If AD= 4cm, DB=4.Scm, AE=6.4cm and EC=7.2cm, find if DE is parallel to BC or not.
Two figures are similar. If the ratio of their perimeters is 8:16. What will be the ratio of the corresponding sides?
Find the scale factor in each of the following and state the type of size transformation:
Actual length = 12cm, Image length = 15cm.
ΔABC ~ ΔDEF. Write the ratios of their corresponding sides
An architecture have model of building. Length of building is 1 m then length of model is 0.75 cm. Then find length and height of model building whose actual length is 22.5 m and height is 10 m
