Advertisements
Advertisements
प्रश्न
In Millikan’s oil drop experiment, what is the terminal speed of an uncharged drop of radius 2.0 × 10–5 m and density 1.2 × 103 kg m–3? Take the viscosity of air at the temperature of the experiment to be 1.8 × 10–5 Pa s. How much is the viscous force on the drop at that speed? Neglect buoyancy of the drop due to air.
उत्तर १
Terminal speed = 5.8 cm/s; Viscous force = 3.9 × 10–10 N
Radius of the given uncharged drop, r = 2.0 × 10–5 m
Density of the uncharged drop, ρ = 1.2 × 103 kg m–3
Viscosity of air, `eta = 1.8 xx 10^(-5) Pa s`
Density of air (`rho_0`) can be taken as zero in order to neglect buoyancy of air.
Acceleration due to gravity, g = 9.8 m/s2
Terminal velocity (v) is given by the relation:
`v = (2r^2 xx (rho - rho_0)g)/(9eta)`
`= (2xx(2.0xx10^(-5))^2 (1.2xx10^3 - 0)xx 9.8)/(9xx1.8xx10^(-5))`
`= 5.807 xx10^(-2) ms^(-1)`
`= 5.8 cm^1`
Hence, the terminal speed of the drop is 5.8 cm s–1.
The viscous force on the drop is given by:
`F = 6pietarv`
`:. F = 6xx3.14xx1.8xx10^(-5)xx2.0xx^(-5)xx 5.8xx10^(-2)`
`= 3.9 xx 10^(-10) N`
Hence, the viscous force on the drop is 3.9 × 10–10 N.
उत्तर २
Here radius of drop, r = 2.0 x 10-5 m, density of drop, p = 1.2 x 103 kg/m3, viscosity of air TI = 1.8 x 10-5Pa-s.
Neglecting upward thrust due to air, we find that terminal speed is
`v_r = 2/9 (r62rhog)/eta = (2xx(2.0xx10^(-5))^2xx(1.2xx10^3))xx9.8)/(9xx(1.8xx10^(-5)))`
`= 5.81 xx 10^(-2) ms^(-1) " or " 5.81 cm s^(-1)`
Viscous force at this speed
`F = 6pietarv = 6 xx 3.14 xx (1.8xx10^(-5))xx (2.0xx10^(-5))xx (5.81xx10^(-2))`
`= 3.94 xx 10^(10) N`
APPEARS IN
संबंधित प्रश्न
(a) What is the largest average velocity of blood flow in an artery of radius 2 × 10–3 m if the flow must remain laminar? (b) What is the corresponding flow rate? (Take viscosity of blood to be 2.084 × 10–3 Pa s).
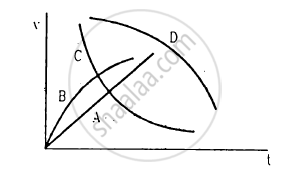
A spherical ball is dropped in a long column of a viscous liquid. The speed of the ball as a function of time may be best represented by the graph
Estimate the speed of vertically falling raindrops from the following data. Radius of the drops = 0.02 cm, viscosity of air = 1.8 × 10−4 poise, g= 9.9 × 10 ms−2 and density of water = 1000 kg m−3.
Which has greater viscosity, oil or honey? Why?
Distinguish between streamlined flow and turbulent flow.
A small metal sphere of mass M and density d1, when dropped in a jar filled with liquid moves with terminal velocity after sometime. The viscous force acting on the sphere is (d2 = density of liquid and g = gravitational acceleration)
With increase in temperature, the viscosity of ______.
- gases decreases.
- liquids increases.
- gases increases.
- liquids decreases.
Define the coefficient of viscosity.
Define velocity gradient.
The dimensions of coefficient of viscosity are ______.
