Advertisements
Advertisements
प्रश्न
In a parallelogram ABCD, if ∠D = 115°, then write the measure of ∠A.
उत्तर
In Parallelogram ABCD , ∠A and are ∠D Adjacent angles.
We know that in a parallelogram, adjacent angles are supplementary.
\[\text { Now}, \angle A + \angle D = 180^\circ \]
\[ \Rightarrow \angle A + 115^\circ = 180^\circ\]
\[ \Rightarrow \angle A = 180^\circ - 115^\circ\]
\[ \Rightarrow \angle A = 65^\circ\]
\[\text{So, measure of } \angle\text { A is } 65^\circ .\]
APPEARS IN
संबंधित प्रश्न
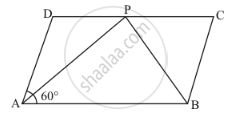
In Fig., below, ABCD is a parallelogram in which ∠A = 60°. If the bisectors of ∠A and ∠B meet at P, prove that AD = DP, PC = BC and DC = 2AD.
ABCD is a square. AC and BD intersect at O. State the measure of ∠AOB.
In a parallelogram ABCD, write the sum of angles A and B.
In a parallelogram ABCD, the bisector of ∠A also bisects BC at X. Find AB : AD.
We get a rhombus by joining the mid-points of the sides of a
The figure formed by joining the mid-points of the adjacent sides of a rhombus is a
ABCD is a parallelogram in which diagonal AC bisects ∠BAD. If ∠BAC = 35°, then ∠ABC =
P is the mid-point of side BC of a parallelogram ABCD such that ∠BAP = ∠DAP. If AD = 10 cm, then CD =
In a quadrilateral ABCD, ∠A + ∠C is 2 times ∠B + ∠D. If ∠A = 140° and ∠D = 60°, then ∠B=
In the given figure, ∠A = 64°, ∠ABC = 58°. If BO and CO are the bisectors of ∠ABC and ∠ACB respectively of ΔABC, find x° and y°
