Advertisements
Advertisements
प्रश्न
In a parallelogram ABCD, the bisector of ∠A also bisects BC at X. Find AB : AD.
उत्तर
Parallelogram ABCD is given as follows:
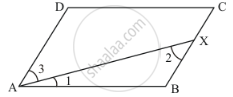
We have AX bisects ∠A bisecting BC at X.
That is, BX = CX
We need to find AB :AD
Since, AX is the bisector ∠A
That is,
`∠1 =1/2 ∠A ` …… (i)
Also, ABCD is a parallelogram
Therefore, AD || BC and AB intersects them
∠A +∠B = 180°
∠B = 180° - ∠A …… (ii)
In ΔABX,by angle sum property of a triangle:
∠1 +∠2 + ∠B = 180°
From (i) and (ii), we get:
`1/2 ∠A + ∠2 + 180° - ∠A = 180°`
`∠2- 1/2 ∠A = 0`
`∠2 = 1/2 ∠A` …… (iii)
From (i) and (iii),we get:
∠1 = ∠2
Sides opposite to equal angles are equal. Therefore,
BX = AB
2BX = 2AB
As X is the mid point of BC. Therefore,
BC = 2AB
Also, ABCD is a parallelogram, then, BC = AD
AD = 2AB
Thus,
AB :AD = AB :2AB
AB :AD = 1:2
Hence the ratio of AB : AD is 1:2.
APPEARS IN
संबंधित प्रश्न
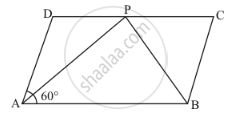
In Fig., below, ABCD is a parallelogram in which ∠A = 60°. If the bisectors of ∠A and ∠B meet at P, prove that AD = DP, PC = BC and DC = 2AD.
In a parallelogram ABCD, determine the sum of angles ∠C and ∠D .
ABCD is a square. AC and BD intersect at O. State the measure of ∠AOB.
The sides AB and CD of a parallelogram ABCD are bisected at E and F. Prove that EBFD is a parallelogram.
In a ΔABC median AD is produced to X such that AD = DX. Prove that ABXC is a
parallelogram.
In a parallelogram ABCD, if ∠D = 115°, then write the measure of ∠A.
We get a rhombus by joining the mid-points of the sides of a
The figure formed by joining the mid-points of the adjacent sides of a parallelogram is a
ABCD is a parallelogram in which diagonal AC bisects ∠BAD. If ∠BAC = 35°, then ∠ABC =
In the given Figure, if AB = 2, BC = 6, AE = 6, BF = 8, CE = 7, and CF = 7, compute the ratio of the area of quadrilateral ABDE to the area of ΔCDF. (Use congruent property of triangles)
