Advertisements
Advertisements
प्रश्न
In the figure, ray AE || ray BD, ray AF is the bisector of ∠EAB and ray BC is the bisector of ∠ABD. Prove that line AF || line BC.

उत्तर
Since, ray AF bisects ∠EAB and ray BC bisects ∠ABD, then
∠EAF = ∠FAB = ∠x = `1/2`∠EAB and
∠CBA = ∠DBC = ∠y = `1/2`∠ABD
∴ ∠x = `1/2`∠EAB and ∠y = `1/2`∠ABD ...(1)
Since ray AE || ray BD and segment AB is a transversal intersecting them at A and B, then
∠EAB = ∠ABD ...(Alternate interior angles)
On multiplying both sides by `1/2`, we get
`1/2"∠EAB" = 1/2"∠ABD"`
Now, using (1), we get
∠x = ∠y
But ∠x and ∠y are alternate interior angles formed by a transversal AB of ray AF and ray BC.
∴ ray AF || ray BC ...(Alternate angles test)
APPEARS IN
संबंधित प्रश्न
In the given figure, y = 108° and x = 71° Are the lines m and n parallel? Justify.

A transversal EF of line AB and line CD intersects the lines at point P and Q respectively. Ray PR and ray QS are parallel and bisectors ∠BPQ and ∠PQC respectively. Prove that line AB || line CD.

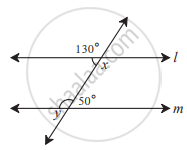
In the given figure, measures of some angles are shown. Using the measures find the measures of ∠x and ∠y and hence show that line l || line m.
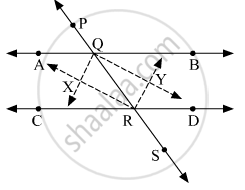
In the given figure, if line AB || line CF and line BC || line ED then prove that ∠ABC = ∠FDE.

In the given figure, line PS is a transversal of parallel line AB and line CD. If Ray QX, ray QY, ray RX, ray RY are angle bisectors, then prove that `square` QXRY is a rectangle.