Advertisements
Advertisements
प्रश्न
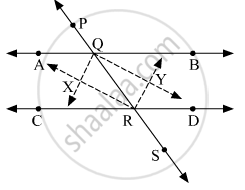
In the given figure, line PS is a transversal of parallel line AB and line CD. If Ray QX, ray QY, ray RX, ray RY are angle bisectors, then prove that `square` QXRY is a rectangle.
उत्तर
Given: AB and CD are two parallel lines which are cut by a transversal PS at the points Q and R respectively. The bisectors of the interior angles intersect at points X and Y.
To prove: Quadrilateral QXRY is a rectangle.
Proof: Since AB || CD and PS is a transversal.
∠AQR = ∠DRQ ...(Alternate interior angles)
⇒ `1/2` ∠AQR = `1/2` ∠DRQ ...(1)
Since QX bisects ∠AQR and RY bisects ∠DRQ, then
∠XQR = `1/2`∠AQR and ∠YRQ = `1/2`∠DRQ
∴ from (1), we get
∠XQR = ∠YRQ
But ∠XQR and ∠YRQ are alternate interior angles formed by the transversal QR with QX and RY respectively.
∴ QX || RY ...(Alternate angles test)
Similarly, we have RX || QY.
Hence, in quadrilateral QXRY, we have QX || RY and RX || QY.
It is known that, a quadrilateral is a parallelogram if its opposite sides are parallel.
∴ QXRY is a parallelogram.
Since sum of the interior angles on the same side of transversal is 180∘, then
∠BQR + ∠DRQ = 180∘
⇒ `1/2` ∠BQR + `1/2` ∠DRQ = 90∘ ...(2)
Since QY bisects ∠BQR and RY bisects ∠DRQ, then
∠YQR = `1/2`∠BQR and ∠YRQ = `1/2`∠DRQ
∴ from (2), we get
∠YQR + ∠YRQ = 90∘ ...(3)
In ∆QRY, we have
∠YQR + ∠YRQ + ∠QYR = 180∘ ...(Angle sum property of triangle)
⇒ 90∘ + ∠QYR = 180∘ ...[Using (3)]
⇒ ∠QYR = 180∘ − 90∘
⇒ ∠QYR = 90∘
Since QXRY is a parallelogram, then
∠QXR = ∠QYR ...(Opposite angles of parallolegram are equal)
⇒ ∠QXR = 90∘ ...(∵ ∠QYR = 90∘)
Since adjacent angles in a parallelogram are supplementary, then
∠QXR + ∠XRY = 180∘
⇒ 90∘ + ∠XRY = 180∘ ...(∵ ∠QXR = 90∘)
⇒ ∠XRY = 180∘ − 90∘
⇒ ∠XRY = 90∘
Also, ∠XQY = ∠XRY = 90∘ ...(Opposite angles of parallolegram are equal)
Thus, QXRY is a parallelogram in which all the interior angles are right angles.
It is known that, a rectangle is a parallolegram in which each angle is a right angle.
Hence, `square` QXRY is a rectangle.
APPEARS IN
संबंधित प्रश्न
In the given figure, y = 108° and x = 71° Are the lines m and n parallel? Justify.

In the figure, ray AE || ray BD, ray AF is the bisector of ∠EAB and ray BC is the bisector of ∠ABD. Prove that line AF || line BC.

A transversal EF of line AB and line CD intersects the lines at point P and Q respectively. Ray PR and ray QS are parallel and bisectors ∠BPQ and ∠PQC respectively. Prove that line AB || line CD.

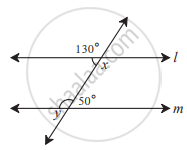
In the given figure, measures of some angles are shown. Using the measures find the measures of ∠x and ∠y and hence show that line l || line m.
In the given figure, if line AB || line CF and line BC || line ED then prove that ∠ABC = ∠FDE.

