Advertisements
Advertisements
प्रश्न
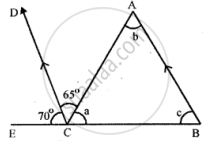
In the following figure, BA is parallel to CD. Find the angles a, b and c:
उत्तर
In the given figure,
ABC is a triangle and AB || DC and AC is its transversal.
∠BAC = ∠ACD (Alternate angles)
⇒ b = 65°
Again AB || DC and BCE is its transversal
∴ ∠ABC = ∠DCE
⇒ C = 70°
But ∠ACB + ∠ACD + ∠DCE = 180 (Straight line angle)
∴ a + 65° + 70° = 180°
⇒ a + 135° = 180°
⇒ a = 180° - 135° = 45°
Hence a = 45°, b = 65° and c = 70°
APPEARS IN
संबंधित प्रश्न
Construct the 60° angle, using ruler and a pair of compass only.
Construct the 30° angle, using ruler and a pair of compass only.
Draw a line segment PQ = 8cm. Construct the perpendicular bisector of the line segment PQ. Let the perpendicular bisector drawn meet PQ at point R. Measure the lengths of PR and QR. Is PR = QR?
Draw a line segment AB = 6.5 cm. Locate a point P that is 5 cm from A and 4.6 cm from B. Through the point P, draw a perpendicular on to the line segment AB.
Draw a line segment OP = 8cm. Use set-square to construct ∠POQ = 90°; such that OQ = 6 cm. Join P and Q; then measure the length of PQ.
In the following figures, PQ is parallel to RS. Find the angles a, b and c:
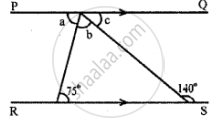
In the following figures, PQ is parallel to RS. Find the angles a, b and c:

Two straight lines are cut by a transversal so that the co-interior angles are supplementary. Are the straight lines parallel?
Two straight lines are cut by a transversal so that the co-interior angles are equal. What must be the measure of each interior angle to make the straight lines parallel to each other?
In the case given below, find the value of x so that POQ is straight line

