Advertisements
Advertisements
प्रश्न
In the given figure, ABC is a triangle in which ∠BAC = 30°. Show that BC is equal to the radius of the circumcircle of the triangle ABC, whose centre is O.
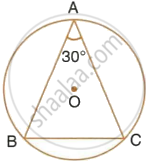
उत्तर
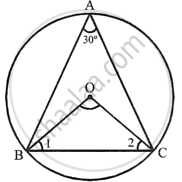
Given – In the figure ABC is a triangle in which ∠A = 30°
To prove – BC is the radius of circumcircle of ∆ABC whose centre is O.
Construction – Join OB and OC.
Proof – ∠BOC = 2∠BAC = 2 × 30° = 60°
Now in ∆OBC,
OB = OC ...[Radii of the same circle]
∠OBC = ∠OCB
But, in ΔBOC,
∠OBC + ∠OCB + ∠BOC = 180° ...[Angles of a triangle]
`=>` ∠OBC + ∠OBC + 60° = 180°
`=>` 2∠OBC + 60° = 180°
`=>` 2∠OBC = 180° – 60°
`=>` 2∠OBC = 120°
`=> ∠OBC = 120^circ/2 = 60^circ`
`=>` ∠OBC = ∠OCB = ∠BOC = 60°
`=>` ΔBOC is an equilateral triangle
`=>` BC = OB = OC
But, OB and OC are the radii of the circumcircle
∴ BC is also the radius of the circumcircle.
APPEARS IN
संबंधित प्रश्न
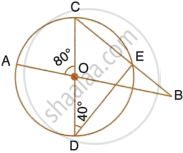
In the figure given alongside, AB and CD are straight lines through the centre O of a circle. If ∠AOC = 80° and ∠CDE = 40°, find the number of degrees in:
- ∠DCE,
- ∠ABC.
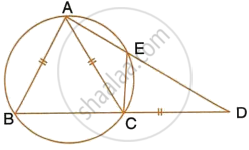
In the given figure, AB = AC = CD and ∠ADC = 38°. Calculate :
- Angle ABC
- Angle BEC
The given figure shows a circle with centre O such that chord RS is parallel to chord QT, angle PRT = 20° and angle POQ = 100°. Calculate:
(ii) angle QRP

The given figure shows a circle with centre O such that chord RS is parallel to chord QT, angle PRT = 20° and angle POQ = 100°. Calculate:
(iii) angle QRS
The given figure shows a circle with centre O such that chord RS is parallel to chord QT, angle PRT = 20° and angle POQ = 100°. Calculate:
(iv) angle STR

In the given figure, an equilateral triangle ABC is inscribed in a circle with center O.
Find: (i) ∠BOC
(ii) ∠OBC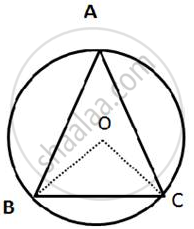
In the given figure, AB is a side of a regular hexagon and AC is a side of a regular eight-sided polygon.
Find:
(i) ∠AOB
(ii) ∠AOC
(iii) ∠BOC
(iv) ∠OBC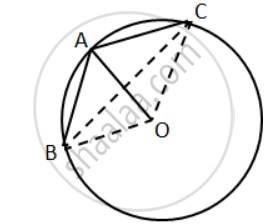
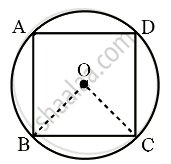
In the given figure, a square is inscribed in a circle with center O. Find:
- ∠BOC
- ∠OCB
- ∠COD
- ∠BOD
Is BD a diameter of the circle?
In the given figure, AB is a side of regular pentagon and BC is a side of regular hexagon.
(i) ∠AOB
(ii) ∠BOC
(iii) ∠AOC
(iv) ∠OBA
(v) ∠OBC
(vi) ∠ABC
In the given figure, arc AB and arc BC are equal in length. If ∠AOB = 48°, find:
(i) ∠BOC
(ii) ∠OBC
(iii) ∠AOC
(iv) ∠OAC
