Advertisements
Advertisements
प्रश्न
In the given figure, PQ is tangent to a circle centred at O and ∠BAQ = 30°, show that BP = BQ.
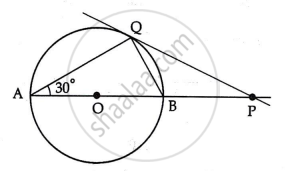
बेरीज
उत्तर
∠BQP = ∠BAQ ...(∠s in alternate segment are equal)
⇒ ∠BQP = 30° ...(∵ ∠BAQ = 30° given) ...(i)
As AB is a diameter, AQB is a Semicircle.
∠AQB = 90° ...(angle in semicircle = 90°)
From Fig. ∠AQP = ∠AQB + ∠BQP
⇒ ∠AQP = 90° + 30°
∠AQP = 120°
In ΔAQP,
∠QPA + ∠BAQ + ∠AQP = 180°
⇒ ∠QPA = 30° + 120°
∠QPA = 180°
⇒ ∠QPA = 180 − (30° +120°)
⇒ ∠QPA = 30° ...(ii)
From (i) and (ii) we get
∠BQP = ∠QPB = 30°
Therefore, QB = BP
shaalaa.com
या प्रश्नात किंवा उत्तरात काही त्रुटी आहे का?
