Advertisements
Advertisements
प्रश्न
In the given figure, seg PT is the bisector of ∠QPR. A line through R intersects ray QP at point S. Prove that PS = PR.

उत्तर
Given: Seg PT is the bisector of ∠QPR.
To prove: PS = PR
Construction: Draw seg SR || seg PT.
Proof:
seg PT is the bisector of ∠QPR. ...[Given]
∴ ∠QPT = ∠RPT ...(i)
seg PT || seg SR ...[Construction]
and seg QS is their transversal.
∴ ∠QPT = ∠PSR …(ii) ...[Corresponding angles]
seg PT || seg SR ...[Construction]
and seg PR is their transversal.
∴ ∠RPT = ∠PRS ...(iii) ...[Alternate angles]
∴ ∠PRS = ∠PSR …(iv) ...[From (i), (ii) and (iii)]
In ∆PSR,
∠PRS = ∠PSR ...[From (iv)]
∴ PS = PR ...[Converse of isosceles triangle theorem]
APPEARS IN
संबंधित प्रश्न
In the given figure, point A is on the bisector of ∠ XYZ. If AX = 2 cm then find AZ.


In the given figure, ∠RST = 56°, seg PT ⊥ ray ST, seg PR ⊥ ray SR and seg PR ≅ seg PT. Find the measure of ∠RSP. State the reason for your answer.
Prove that, if the bisector of ∠BAC of ΔABC is perpendicular to side BC, then ΔABC is an isosceles triangle.
In ΔPQR, If PQ > PR and bisectors of ∠Q and ∠R intersect at S. Show that SQ > SR.

In the figure, point D and E are on side BC of ΔABC, such that BD = CE and AD = AE. Show that ΔABD ≅ ΔACE.
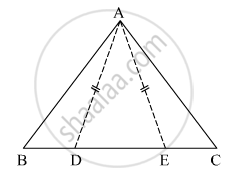
In the given figure, bisector of ∠BAC intersects side BC at point D. Prove that AB > BD.

In the given figure, seg AD ⊥ seg BC. seg AE is the bisector of ∠CAB and C - E - D. Prove that ∠DAE = `1/2` (∠C - ∠B)