Advertisements
Advertisements
प्रश्न
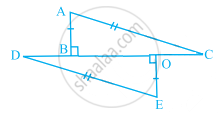
In the given pairs of triangles of the figure, using only RHS congruence criterion, determine which pairs of triangles are congruent. In congruence, write the result in symbolic form:
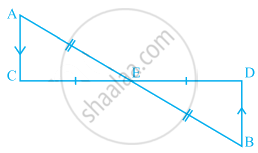
उत्तर
In ∆ACE and ∆BDE,
∠ACE = ∠BDE ......(AC || BD, alternate interior angles)
CE = DE ......(Given)
∠AEC = ∠BED ......(Vertically opposite angles)
∴ ∆ACE ≅ ∆BDE ......(ASA criterion)
∴ Triangles are congruent but not by RHS congruence criterion
APPEARS IN
संबंधित प्रश्न
Given below are measurements of some parts of two triangles. Examine whether the two triangles are congruent or not, using the RHS congruence rule. In the case of congruent triangles, write the result in symbolic form:
∆ABC, ∠B = 90°, AC = 8 cm, AB = 3 cm.
∆PQR, ∠P = 90°, PR = 3 cm, QR = 8 cm.
Given below are measurements of some parts of two triangles. Examine whether the two triangles are congruent or not, using the RHS congruence rule. In the case of congruent triangles, write the result in symbolic form:
∆ABC, ∠A = 90°, AC = 5 cm, BC = 9 cm.
∆PQR, ∠Q = 90°, PR = 8 cm, PQ = 5 cm.
In Fig, DA ⊥ AB, CB ⊥ AB, and AC = BD. State the three pairs of equal parts in ∆ABC and ∆DAB. Which of the following statements is meaningful?
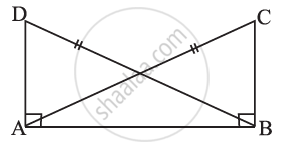
(i) ∆ABC ≅ ∆BAD
(ii) ∆ABC ≅ ∆ABD.
In Fig,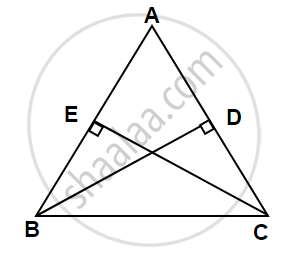
BD and CE are altitudes of ∆ABC such that BD = CE.
(i) State the three pairs of equal parts in ∆CBD and ∆BCE.
(ii) Is ∆CBD ≅ ∆BCE? Why or why not?
(iii) Is ∠DCB = ∠EBC? Why or why not?
In the given figure, ∠CIP ≡ ∠COP and ∠HIP ≡ ∠HOP. Prove that IP ≡ OP.
In the given figure, D is the midpoint of OE and ∠CDE = 90°. Prove that ΔODC ≡ ΔEDC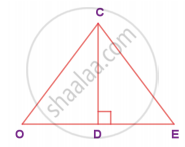
For the given pair of triangles state the criterion that can be used to determine the congruency?
In the given pairs of triangles of the figure, using only RHS congruence criterion, determine which pairs of triangles are congruent. In congruence, write the result in symbolic form:

In the given pairs of triangles of the figure, using only RHS congruence criterion, determine which pairs of triangles are congruent. In congruence, write the result in symbolic form:
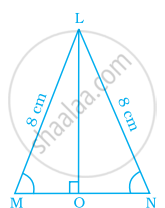
In the given figure, state the three pairs of equal parts in ΔABC and ΔEOD. Is ΔABC = ΔEOD? Why?