Advertisements
Advertisements
प्रश्न
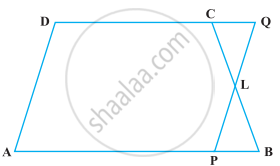
In trapezium ABCD, AB || DC and L is the mid-point of BC. Through L, a line PQ || AD has been drawn which meets AB in P and DC produced in Q (Figure). Prove that ar (ABCD) = ar (APQD)
उत्तर
Given: In trapezium ABCD, AB || DC, DC produced in Q and L is the mid-point of BC.
∴ BL = CL
To prove: ar (ABCD) = ar (APQD)
Proof: Since, DC produced in Q and AB || DC.
So, DQ || AB
In ΔCLQ and ΔBLP,
CL = BL ...[Since, L is the mid-point of BC]
∠LCQ = ∠LBP ...[Alternate interior angles as BC is a transversal]
∠CQL = ∠LPB ...[Alternate interior angles as PQ is a transversal]
∴ ΔCLQ ≅ ΔBLP ...[By AAS congruence rule]
Then, ar (ΔCLQ) = ar (ΔBLP) [Since, congruent triangles have equal area] ...(i)
Now, ar (ABCD) = ar (APQD) – ar (ΔCQL) + ar (ΔBLP)
= ar (APQD) – ar (ΔBLP) + ar (ΔBLP) ...[From equation (i)]
⇒ ar (ABCD) = ar (APQD)
Hence proved.
APPEARS IN
संबंधित प्रश्न
If E, F, G and H are respectively the mid-points of the sides of a parallelogram ABCD show that ar (EFGH) = 1/2ar (ABCD)
P and Q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar (APB) = ar (BQC).
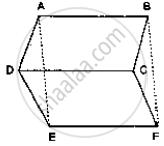
In the given below fig. ABCD, ABFE and CDEF are parallelograms. Prove that ar (ΔADE)
= ar (ΔBCF)
In which of the following figures, you find two polygons on the same base and between the same parallels?
PQRS is a rectangle inscribed in a quadrant of a circle of radius 13 cm. A is any point on PQ. If PS = 5 cm, then ar (PAS) = 30 cm2.
In the following figure, PSDA is a parallelogram. Points Q and R are taken on PS such that PQ = QR = RS and PA || QB || RC. Prove that ar (PQE) = ar (CFD).

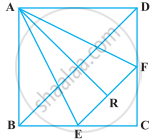
ABCD is a square. E and F are respectively the mid-points of BC and CD. If R is the mid-point of EF (Figure), prove that ar (AER) = ar (AFR)
ABCD is a parallelogram in which BC is produced to E such that CE = BC (Figure). AE intersects CD at F. If ar (DFB) = 3 cm2, find the area of the parallelogram ABCD.
If the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram so formed will be half of the area of the given quadrilateral (Figure).
[Hint: Join BD and draw perpendicular from A on BD.]

ABCD is a trapezium in which AB || DC, DC = 30 cm and AB = 50 cm. If X and Y are, respectively the mid-points of AD and BC, prove that ar (DCYX) = `7/9` ar (XYBA)
