Advertisements
Advertisements
प्रश्न
In triangle ABC, D is a point in AB such that AC = CD = DB. If ∠B = 28°, find the angle ACD.
उत्तर

ΔDBC is an isosceles triangle.
As, Side CD = Side DB
⇒ ∠DBC = ∠DCB ...[If two sides of a triangle are equal, then angles opposite to them are equal]
And ∠B = ∠DBC = ∠DCB = 28°
As the sum of all the angles of the triangle is 180°
∠DCB + ∠DBC + ∠BCD = 180°
⇒ 28° + 28° + ∠BCD = 180°
⇒ ∠BCD = 180° − 56°
⇒ ∠BCD = 124°
Sum of two non-adjacent interior angles of a triangle is equal to the exterior angle.
⇒ ∠DBC+ ∠DCB = ∠ADC
⇒ 28° + 28° = ∠ADC
⇒ ∠ADC = 56°
Now ΔACD is an isosceles triangle with AC = DC
⇒ ∠ADC = ∠DAC = 56°
Sum of all the angles of a triangle is 180°
⇒ ∠ACD + ∠ADC + ∠DAC = 180°
⇒ ∠ACD + 56° + 56° = 180°
⇒ ∠ACD = 180° − 112°
⇒ ∠ACD = 68°
APPEARS IN
संबंधित प्रश्न
Find the values of x and y using the information shown in the figure.

Find the measure of ∠ABD and m∠ACD.
ΔABC is isosceles in which AB = AC. Seg BD and seg CE are medians. Show that BD = CE.
ABC is an equilateral triangle. Its side BC is produced up to point E such that C is mid-point of BE. Calculate the measure of angles ACE and AEC.
In triangle ABC; angle ABC = 90o and P is a point on AC such that ∠PBC = ∠PCB.
Show that: PA = PB.
In triangle ABC; ∠A = 60o, ∠C = 40o, and the bisector of angle ABC meets side AC at point P. Show that BP = CP.
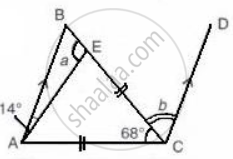
Using the information given of the following figure, find the values of a and b. [Given: CE = AC]
In triangle ABC, AB = AC; BE ⊥ AC and CF ⊥ AB.
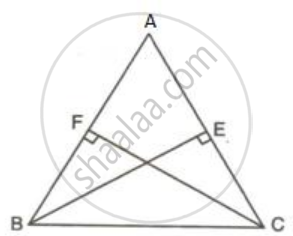
Prove that:
(i) BE = CF
(ii) AF = AE
Through any point in the bisector of an angle, a straight line is drawn parallel to either arm of the angle. Prove that the triangle so formed is isosceles.
If the bisector of an angle of a triangle bisects the opposite side, prove that the triangle is isosceles.
