Advertisements
Advertisements
प्रश्न
In two concentric circles, the radii are OA = r cm and OQ = 6 cm, as shown in the figure. Chord CD of larger circle is a tangent to smaller circle at Q. PA is tangent to larger circle. If PA = 16 cm and OP = 20 cm, the length CD.
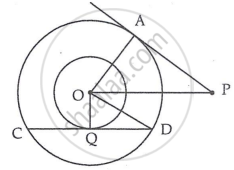
उत्तर
Given that,
OA = r cm, OQ = 6 cm,
PA = 16 cm, OP = 20 cm

In `triangle`AOP,
We have, `angle`OAP = 90° ...[Radius is perpendicular to tangent at point of contact]
So, OP2 = OA2 + AP2
⇒ (20)2 = r2 + (16)2
⇒ r2 = 400 − 256
⇒ r2 = 144
⇒ r = 12cm
Also, OA = OD = 12 cm ...[Radius of circle]
In `triangle`QOD,
We have, `angle`OQD = 90° ...[Radius is perpendicular to tangent at point of contact]
So, OD2 = OQ2 + QD2
⇒ (12)2 = (6)2 + (QD)2
⇒ QD2 = 144 − 36
⇒ QD2 = 108
⇒ QD = `6sqrt3` cm
Also, CD = 2QD ...[As chord is bisected at the point of contact of circle]
So, CD = `2 × 6sqrt3`
= `12sqrt3` cm
Hence, the length of CD is `12sqrt3` cm.
