Advertisements
Advertisements
प्रश्न
(n – 1) equal point masses each of mass m are placed at the vertices of a regular n-polygon. The vacant vertex has a position vector a with respect to the centre of the polygon. Find the position vector of centre of mass.
उत्तर
The centre of mass of a regular polygon with n sides lies on its geometric centre. If mass m is placed at all the n vertices, then the C.O.M Is again at the geometric centre. Let `vecr` be the position vector of the COM and `vecA` of the vacant vertex. Then
`r_cm = ((n - 1)mr + ma)/((n - 1)m + m)` = 0 .....(when mass is placed at nth vertex also)
`(n - 1)mr + ma` = 0
`r = - (ma)/((n - 1)m)`
`vecr = - veca/((n - 1))`
The negative sign depicts that the C.O.M lies on the opposite side of nth vertex.
Hence the center of mass of n particles is a weighted average of the position vectors of n particles making up the system.
The centre of mass of a regular n-polygon lies at its geometrical centre.
Let the position vector of each centre of mass or regular n polygon is r.
(n – 1) equal point masses each of mass m are placed at (n – 1) vertices of the regular n-polygon, therefore, for its centre of mass.
APPEARS IN
संबंधित प्रश्न
Give the location of the centre of mass of a
- sphere,
- cylinder,
- ring, and
- cube,
each of uniform mass density. Does the centre of mass of a body necessarily lie inside the body?
If all the particle of a system lie in a cube, is it necessary that the centre of mass be in the cube?
Consider the following the equations
(A) \[\vec{R} = \frac{1}{M} \sum_i m_i \vec{r_i}\] and
(B) \[\vec{a}_{CM} = \frac{\vec{F}}{M}\]
In a noninertial frame
In which of the following cases the centre of mass of a rod is certainly not at its centre?
(a) the density continuously increases from left to right
(b) the density continuously decreases from left to right
(c) the density decreases from left to right upto the centre and then increases
(d) the density increases from left to right upto the centre and then decreases.
If the external force acting on a system have zero resultant, the centre of mass
(a) must not move
(b) must not accelerate
(c) may move
(d) may accelerate.
Seven homogeneous bricks, each of length L, are arranged as shown in figure. Each brick is displaced with respect to the one in contact by L/10. Find the x-coordinate fo the centre of mass relative to the origin shown.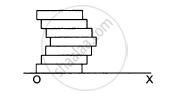
Two fat astronauts each of mass 120 kg are travelling in a closed spaceship moving at a speed of 15 km/s in the outer space far removed from all other material objects. The total mass of the spaceship and its contents including the astronauts is 660 kg. If the astronauts do slimming exercise and thereby reduce their masses to 90 kg each, with what velocity will the spaceship move?
A block of mass 2.0 kg moving 2.0 m/s collides head on with another block of equal mass kept at rest. (a) Find the maximum possible loss in kinetic energy due to the collision. (b) If he actual loss in kinetic energy is half of this maximum, find the coefficient of restitution.
The centre of mass of a system of two particles divides the distance between them ______.
A point charge Q is situated at point B on the ground. A point charge q of mass m is vertically dropped along line AB from a multi-storey building of height h. Find the position of the point charge q when it is in equilibrium.

