Advertisements
Advertisements
प्रश्न
One angle of a triangle is 60°. The other two angles are in the ratio of 5: 7. Find the two angles.
Advertisements
उत्तर
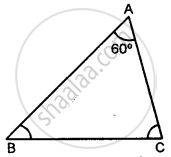
In Δ ABC,
Let ∠A = 60° and then ∠B: ∠C = 5: 7
But ∠A + ∠B + ∠C = 180° ........(Angles of a triangle)
⇒ 60° + ∠B + ∠C = 180°
⇒ ∠B + ∠C = 180°− 60° = 120°
Let ∠B = 5x and ∠C = 7x
∴ 5x + 7x = 120°
⇒ 12x = 120°
⇒ x =`(120°)/12=10°`
∴ ∠B = 5x = 5 × 10° = 50°
∠C = 7x = 7 × 10° = 70°
APPEARS IN
संबंधित प्रश्न
In a ΔABC, if ∠A = 55°, ∠B = 40°, find ∠C.
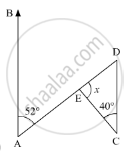
In the given figure, AB divides ∠DAC in the ratio 1 : 3 and AB = DB. Determine the value of x.
If the side BC of ΔABC is produced on both sides, then write the difference between the sum of the exterior angles so formed and ∠A.
If the angles of a triangle are equal, find its angles.
In the following, find the marked unknown angle:
Can a triangle together have the following angles?
85°, 95° and 22°
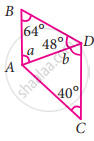
Find, giving a reason, the unknown marked angles, in a triangle drawn below:
As shown in the figure, Avinash is standing near his house. He can choose from two roads to go to school. Which way is shorter? Explain why.
The exterior angle of a triangle is equal to the sum of two
Prove that in a triangle, other than an equilateral triangle, angle opposite the longest side is greater than `2/3` of a right angle.
