Advertisements
Advertisements
प्रश्न
Calculate the angles of a triangle if they are in the ratio 4: 5: 6.
उत्तर
We know that sum of angles of a triangle is 180°
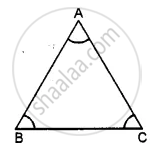
∴ ∠A + ∠B + ∠C = 180°
But ∠A: ∠B: ∠C = 4: 5: 6
Let ∠A = 4x, ∠B = 5x and ∠C = 6x,
then 4x + 5x + 6x = 180°
⇒ 15x = 180°
⇒ x = `(180°)/15=12°`
∴ ∠A = 4x = 4 × 12° = 48°
∠B = 5x = 5 × 12° = 60°
∠C = 6x = 6 × 12° = 72°
APPEARS IN
संबंधित प्रश्न
If one angle of a triangle is equal to the sum of the other two, show that the triangle is a
right triangle.
Can a triangle have two obtuse angles? Justify your answer in case.
Is the following statement true and false :
All the angles of a triangle can be greater than 60°.
Fill in the blank to make the following statement true:
Sum of the angles of a triangle is ....
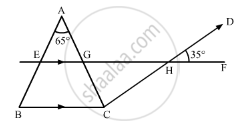
In the given figure, if AB || CD, EF || BC, ∠BAC = 65° and ∠DHF = 35°, find ∠AGH.
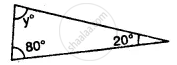
Calculate the unknown marked angles of the following figure :
In the given figure, express a in terms of b.
The length of the three segments is given for constructing a triangle. Say whether a triangle with these sides can be drawn. Give the reason for your answer.
15 cm, 20 cm, 25 cm
Match the following:
| Column A | Column B |
| (i) No sides are equal | Isosceles triangle |
| (ii) One right angle | Scalene triangle |
| (iii) One obtuse angle | Right angled triangle |
| (iv) Two sides of equal length | Equilateral triangle |
| (v) All sides are equal | Obtuse angled triangle |
Identify three triangles in the figure.