Advertisements
Advertisements
Question
Calculate the angles of a triangle if they are in the ratio 4: 5: 6.
Solution
We know that sum of angles of a triangle is 180°
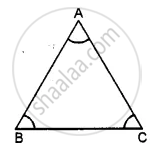
∴ ∠A + ∠B + ∠C = 180°
But ∠A: ∠B: ∠C = 4: 5: 6
Let ∠A = 4x, ∠B = 5x and ∠C = 6x,
then 4x + 5x + 6x = 180°
⇒ 15x = 180°
⇒ x = `(180°)/15=12°`
∴ ∠A = 4x = 4 × 12° = 48°
∠B = 5x = 5 × 12° = 60°
∠C = 6x = 6 × 12° = 72°
APPEARS IN
RELATED QUESTIONS
Determine the measure of each of the equal angles of a right-angled isosceles triangle.
OR
ABC is a right-angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
In the given figure, AC ⊥ CE and ∠A : ∠B : ∠C = 3 : 2 : 1, find the value of ∠ECD.

Fill in the blank to make the following statement true:
An exterior angle of a triangle is always ......... than either of the interior opposite angles.
Define a triangle.
Find x, if the angles of a triangle is:
x°, x°, x°
Classify the following triangle according to sides:
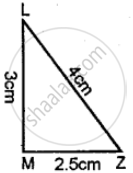
Classify the following triangle according to sides:

The length of the sides of the triangle is given. Say what types of triangles they are 3.4 cm, 3.4 cm, 5 cm.
Can 30°, 60° and 90° be the angles of a triangle?
Can we have two acute angles whose sum is a reflex angle? Why or why not?
