Question

PQR  is an isosceles triangle . If two of its vertices are P (2 , 0) and Q (2 , 5) , find the coordinates of R if the length of each of the two equal sides is 3.

Answer 1

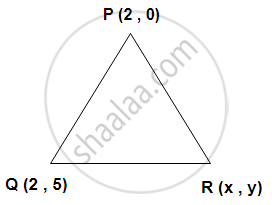
PQ = c

∴ PR = QR = 3 units

Let the coordinates of R be on ,

PR = `sqrt (("x" - 2)^2 + ("y" - 0)^2)` 

`=> 3 = sqrt ("x"^2 + 4 - 4"x" + "y"^2)`

squaring both sides ,

`=> 9 = "x"^2 - 4"x" + "y"^2 + 4`

`=> "x"^2 - 4"x" + "y"^2 - 5 = 0`

`=> "x"^2 + "y"^2 - 4"x" = 5`       .....(1)

QR = `sqrt (("x" - 2)^2 + ("y" - 5)^2)`

`=> 3 = sqrt ("x"^2 + 4 - 4"x" + "y"^2 + 25 - 10"y")`

⇒ 9 = x² + y² - 4x - 10y + 29

⇒ 0 = x² + y² - 4x - 10y + 29

From (1)  0 =   5 - 10y + 20

10 y = 25

y = `5/2`

`=> "x"^2 + 25/4 - 4"x" - 5 = 0`

`=> 4"x"^2 + 25 - 16"x" - 20 = 0`

`=> 4"x"^2 - 16"x" + 5 = 0`

D = (-16)² - 4(4)(5)

= 256 - 80

= 176

`sqrt "d" = sqrt 176 = 4 sqrt 11`

x = `(16 +- 4 sqrt 11)/(2 xx 4)`

= `(4 +- 4 sqrt 11)/2`

= `2 + sqrt 11/2 , 2 - sqrt 11/2`

The coordinates of R are `(2 - sqrt 11/2 , 5/2)` or `(2 + sqrt 11/2 , 5/2)`