Advertisements
Advertisements
प्रश्न
Prove that the points A(-5, 4), B(-1, -2) and C(S, 2) are the vertices of an isosceles right-angled triangle. Find the coordinates of D so that ABCD is a square.
उत्तर
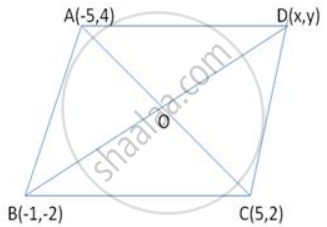
AB = `sqrt ((-1 +5)^2 (-2-4)^2) = sqrt (16+36) = sqrt 52` units
BC = `sqrt ((-1 - 5)^2 + (-2-2)^2) = sqrt (36 + 36) = sqrt 52` units
AC = `sqrt ((5 + 5)^2 + (2 - 4)^2) = sqrt (100 + 4) = sqrt 104` units
AB2 + BC2 = 52 + 52 = 104
AC2 = 104
∵ AB = AC and AB2 + BC2 = AC2
∴ ABC is an isosceles right angled triangle.
Let the coordinates of D be (x , y)
If ABCD is a square ,
Midpoint of AC = mid point of BD
O`((-5 + 5)/2 , (4 + 2)/2) = "O" (("x" - 1)/2 , ("y" - 2)/2)`
O = `("x" - 1)/2 , 3 = ("y" - 2)/2`
x = 1 , y = 8
Coordinates of D are (1 , 8)
APPEARS IN
संबंधित प्रश्न
P(1, -2) is a point on the line segment A(3, -6) and B(x, y) such that AP : PB is equal to 2 : 3. Find the coordinates of B.
In what ratio is the line joining (2, –3) and (5, 6) divided by the x-axis?
In what ratio does the point (1, a) divide the join of (–1, 4) and (4, –1)? Also, find the value of a.
Find the image of the point A(5, –3) under reflection in the point P(–1, 3).
Find the image of the point A(5,3) under reflection in the point P(-1,3).
Find the image of the point A(5,3) under reflection in the point P(-1,3).
Determine the ratio in which the line 3x + y – 9 = 0 divides the line joining (1, 3) and (2, 7).
The midpoint of the line segment AB shown in the diagram is (4, – 3). Write down the coordinates of A and B.
Determine the centre of the circle on which the points (1, 7), (7 – 1), and (8, 6) lie. What is the radius of the circle?
From the adjacent figure:
(i) Write the coordinates of the points A, B, and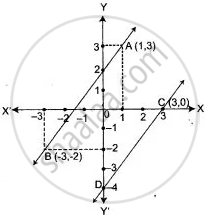
(ii) Write the slope of the line AB.
(iii) Line through C, drawn parallel to AB, intersects Y-axis at D. Calculate the co-ordinates of D.
