Advertisements
Advertisements
प्रश्न
Solve the following equations graphically :
x - 2y = 2
`x/(2) - y` = 1
उत्तर
x - 2y = 2
`x/(2) - y` = 1
x - 2y = 2 ________(1)
`x/(2) - y` = 1 _________(2)
x - 2y = 2
⇒ x = 2 + 2y
Corresponding values of x and y can be tabulated as :
| x | 2 | 0 | 4 |
| y | 0 | -1 | 1 |
Again, `x/(2) - y` = 1
⇒ y = `x/(2) - 1`
Corresponding values of x and y can be tabulated as :
| x | 0 | 2 | 4 |
| y | -1 | 0 | 1 |
Plotting points (0, -1), (2, 0), (4, 1) and joining them, we get a line l1 which is the graph for both the equation (1) and (2).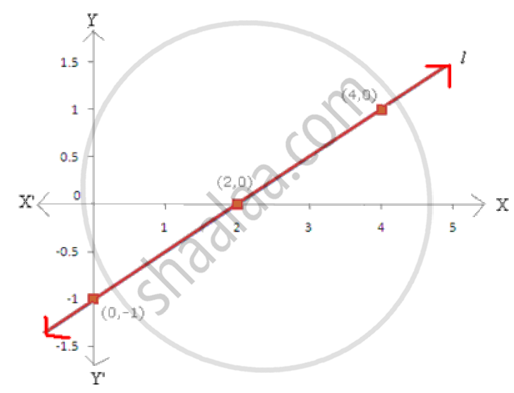
Hence, the given system of equations has infinitely many solutions.
APPEARS IN
संबंधित प्रश्न
Solve graphically the simultaneous equations given below. Take the scale as 2 cm = 1 unit on both the axes.
x - 2y - 4 = 0
2x + y = 3
Find graphically, the vertices of the triangle whose sides have the equations 2y - x = 8; 5y - x = 14 and y - 2x = 1 respectively. Take 1 cm = 1 unit on both the axes.
Use the graphical method to find the value of 'x' for which the expressions `(3x + 2)/(2) and (3)/(4)x - 2`
Solve the following equations graphically :
x + 3y = 8
3x = 2 + 2y
Solve the following equations graphically :
2x + 4y = 7
3x + 8y = 10
Find graphically the vertices of the triangle, whose sides have the equations 2y - x = 8, 5y -x = 14 and y = 2x - 1.
Solve the following system of linear equations graphically :
4x - 5y - 20 = 0
3x + 3y - 15 = 0
Determine the vertices of the triangle formed by the lines, represented by the above equations and the y-axis.
Solve the following system of equations graphically:
6x - 3y + 2 = 7x + 1
5x + 1 = 4x - y + 2
Also, find the area of the triangle formed by these lines and x-axis in each graph.
Solve graphically
`x/2 + y/4` = 1, `x/2 + y/4` = 2
Solve graphically
x = −3, y = 3
