Advertisements
Advertisements
प्रश्न
Solve the following system of inequalities graphically.
2x – y ≥ 1, x – 2y ≤ – 1
उत्तर
To find graphical solution, construct the table as follows:
| Inequation | Equation | Double Intercept form |
Points (x, y) |
Region |
| 2x – y ≥ 1 | 2x – y = 1 |
`(2"x")/1 - "y"/1` = 1 i.e., `"x"/(1/2) + "y"/(-1)` = 1 |
A `(1/2, 0)` B (0, – 1) |
2(0) – 0 `≱ ` 1 ∴ 0 `≱ ` 1 ∴ non-origin side |
| x – 2y ≤ – 1 | x – 2y = – 1 |
`"x"/(-1) - (2"y")/(-1)` = 1 i.e., `"x"/(-1) + "y"/((1/2))` = 1 |
C (– 1, 0), D `(0, 1/2)` |
0 – 2(0) `≰ ` – 1 ∴ 0 `≰` – 1 ∴ non-origin side |
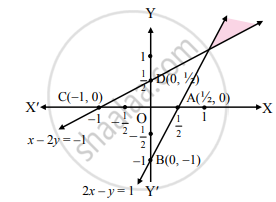
The shaded portion represents the graphical solution.
APPEARS IN
संबंधित प्रश्न
Solve the following system of inequalities graphically: 2x – y > 1, x – 2y < –1
Solve the following system of inequalities graphically: x + y ≤ 6, x + y ≥ 4
Solve the following system of inequalities graphically: 2x + y≥ 8, x + 2y ≥ 10
Solve the following system of inequalities graphically: 5x + 4y ≤ 20, x ≥ 1, y ≥ 2
Solve the following system of inequalities graphically: x – 2y ≤ 3, 3x + 4y ≥ 12, x ≥ 0, y ≥ 1
Solve the following system of inequalities graphically: 4x + 3y ≤ 60, y ≥ 2x, x ≥ 3, x, y ≥ 0
Find all pairs of consecutive odd natural number, both of which are larger than 10, such that their sum is less than 40.
A solution of 8% boric acid is to be diluted by adding a 2% boric acid solution to it. The resulting mixture is to be more than 4% but less than 6% boric acid. If there are 640 litres of the 8% solution, how many litres of 2% solution will have to be added?
Write the set of values of x satisfying the inequation (x2 − 2x + 1) (x − 4) < 0.
Write the set of values of x satisfying |x − 1| ≤ 3 and |x − 1| ≥ 1.
Find the graphical solution of the following system of linear inequations:
x – y ≤ 0, 2x – y ≥ − 2
Find the graphical solution of the following system of linear inequations:
3x + 2y ≤ 1800, 2x + 7y ≤ 1400
Solve the following system of inequalities `(2x + 1)/(7x - 1) > 5, (x + 7)/(x - 8) > 2`
Find the linear inequalities for which the shaded region in the given figure is the solution set.