Advertisements
Advertisements
प्रश्न
The area of a rectangular field is 48 m2 and one of its sides is 6 m. How long will a lady take to cross the field diagonally at the rate of 20 m/minute?
उत्तर
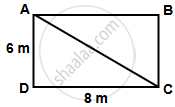
Given, the area of a rectangular field is 48 m2 and one side of the rectangle = 6 m
∴ Area of a rectangle = Length × Breadth
⇒ 48 = 6 × Breadth
⇒ Breadth = 8 m
In ΔACD, ∠D = 90°
So, it is a right-angled triangle.
By using Pythagoras theorem, we have
(AC)2 = (AD)2 + (DC)2
⇒ (AC)2 = (6)2 + (8)2
⇒ (AC)2 = 36 + 64
⇒ `AC = sqrt(100)`
∴ AC = 10 m
Time taken by lady to cross the field diagonally at rate of 20 m/min = `"Distance"/"Speed"`
= `10/20`
= `1/2` min or 30 sec
APPEARS IN
संबंधित प्रश्न
Each side of a rhombus is 18 cm. If the distance between two parallel sides is 12 cm, find its area.
The diagonals of a rhombus are 18 cm and 24 cm. Find:
(i) its area ;
(ii) length of its sides.
(iii) its perimeter
A thin metal iron-sheet is rhombus in shape, with each side 10 m. If one of its diagonals is 16 m, find the cost of painting both sides at the rate of ₹ 6 per m2. Also, find the distance between the opposite sides of this rhombus.
Find the area of rhombus PQRS shown in the following figure.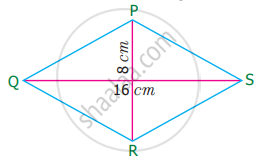
Find the missing value.
| Diagonal (d1) | Diagonal (d2) | Area |
| 26 m | 468 sq.m |
The angle between the diagonals of a rhombus is
A man has to build a rhombus shaped swimming pool. One of the diagonal is 13 m and the other is twice the first one. Then find the area of the swimming pool and also find the cost of cementing the floor at the rate of ₹ 15 per sq.cm
The figure ABCD is a quadrilateral in which AB = CD and BC = AD. Its area is ______.

If the diagonals of a rhombus get doubled, then the area of the rhombus becomes ______ its original area.
The walls and ceiling of a room are to be plastered. The length, breadth and height of the room are 4.5 m, 3 m, and 350 cm respectively. Find the cost of plastering at the rate of Rs 8 per m2.
