Advertisements
Advertisements
प्रश्न
The dining-hall of a hotel is 75 m long; 60 m broad and 16 m high. It has five – doors 4 m by 3 m each and four windows 3 m by 1.6 m each. Find the cost of :
(i) papering its walls at the rate of Rs.12 per m2;
(ii) carpetting its floor at the rate of Rs.25 per m2.
उत्तर
Length of the dining hall of a hotel = 75 m
The breadth of the dining hall of a hotel = 60 m
Height of the dining hall of a hotel = 16 m
(i) Area of four walls of the dining hall = 2[L+B) x H = 2(75 + 60) x 16
= `2(135) xx 16`
= `270 xx 16`
= 4320 m2
Area of one door = `4 xx 3` m2
= 12 m2
Area of 5 doors = `12 xx 5 = 60` m2
Area of one window = `3 xx 1.6 = 4.8`m2
Area of 4 window = `4.8 xx 4 = 19.2`m2
Area of the walls to be papered
= 4320 - (60 + 19.2)
= 4320 - 79.2
= 4240.8 m2
Cost of papering the walls @ Rs.12 per m2
= `4240.8 xx 12`
= Rs. 50889.60
(ii) Area of floor = L × B
= `75 xx 60`
= 4500 m2
Cost of carpetting the floor @ Rs. 25 per m2
= 4500 × 25
= Rs. 112500
APPEARS IN
संबंधित प्रश्न
The floor of a rectangular hall has a perimeter 250 m. If the cost of panting the four walls at the rate of Rs.10 per m2 is Rs.15000, find the height of the hall.
[Hint: Area of the four walls = Lateral surface area.]
Hameed has built a cubical water tank with lid for his house, with each other edge 1 .5 m long. He gets the outer surface of the tank excluding the base, covered with square tiles of side 25 cm. Find how much he would spend for the tiles, if the cost of tiles is Rs. 360 per dozen.
Each edge of a cube is increased by 50%. Find the percentage increase in the surface area of the cube.
Ravish wanted to make a temporary shelter for his car by making a box-like structure with tarpaulin that covers all the four sides and the top of the car ( with the front face as a flap which can be rolled up). Assuming that the stitching margins are very small, and therefore negligible, how much tarpaulin would be required to make the shelter of height 2.5 m with
base dimensions 4 m × 3m?
Find the number of cuboidal boxes measuring 2 cm by 3 cm by 10 cm which can be stored in a carton whose dimensions are 40 cm, 36 cm and 24 cm.
How many soap cakes can be placed in a box of size 56 cm × 0.4 m × 0.25 m, if the size of a soap cake is 7 cm × 5 cm × 2.5 cm?
A village, having a population of 4000, requires 150 litres water per head per day. It has a tank which is 20 m long, 15 m broad and 6 m high. For how many days will the water of this tank last?
A cuboidal box is 5 cm by 5 cm by 4 cm. Find its surface area.
A cloassroom is 11 m long, 8 m wide and 5 m high. Find the sum of the areas of its floor and the four walls (including doors, windows, etc.)
A swimming pool is 20 m long 15 m wide and 3 m deep. Find the cost of repairing the floor and wall at the rate of Rs 25 per square metre.
Show that the product of the areas of the floor and two adjacent walls of a cuboid is the square of its volume.
Three cubes of metal whose edges are in the ratio 3 : 4 : 5 are melted down in to a single cube whose diagonal is 12 `sqrt(3)` cm. Find the edges of three cubes.
The surface area of a cuboid is 1300 cm2. If its breadth is 10 cm and height is 20 cm2, find its length.
The area of the floor of a room is 15 m2. If its height is 4 m, then the volume of the air contained in the room is
The number of cubes of side 3 cm that can be cut from a cuboid of dimensions 10 cm × 9 cm × 6 cm, is ______.
Total surface area of a box of cuboid shape is 500 sq. unit. Its breadth and height is 6 unit and 5 unit respectively. What is the length of that box ?
The volume of a cuboid is 3456 cm3. If its length = 24 cm and breadth = 18 cm ; find its height.
The breadth and height of a rectangular solid are 1.20 m and 80 cm respectively. If the volume of the cuboid is 1.92 m3; find its length.
Find the length of each edge of a cube, if its volume is :
(i) 216 cm3
(ii) 1.728 m3
A closed box is cuboid in shape with length = 40 cm, breadth = 30 cm and height = 50 cm. It is made of a thin metal sheet. Find the cost of metal sheet required to make 20 such boxes, if 1 m2 of metal sheet costs Rs. 45.
A closed box measures 66 cm, 36 cm and 21 cm from outside. If its walls are made of metal-sheet, 0.5 cm thick; find :
(i) the capacity of the box ;
(ii) the volume of metal-sheet and
(iii) weight of the box, if 1 cm3 of metal weighs 3.6 gm.
The height of a circular cylinder is 20 cm and the diameter of its base is 14 cm. Find:
(i) the volume
(ii) the total surface area.
A cuboid is 8 m long, 12 m broad and 3.5 high, Find its
(i) total surface area
(ii) lateral surface area
A room is 22m long, 15m broad and 6m high. Find the area of its four walls and the cost of painting including doors and windows at the rate of Rs.12per m2.
A room is 5m long, 2m broad and 4m high. Calculate the number of persons it can accommodate if each person needs 0.16m3 of air.
Opposite faces of a cuboid are ______ in area.
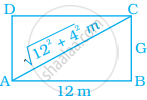
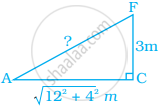
Find the length of the largest pole that can be placed in a room of dimensions 12 m × 4 m × 3 m.