Question

The following figure shows a part of an electric circuit. The potentials at the points a, b and care 30 V, 12 V and 2 V respectively. Find the currents through the three resistors.

Answer 1

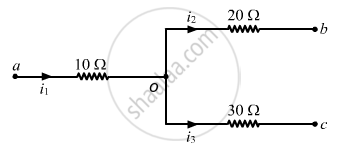
Let the potential at the point o be X volts.

From the figure,

\[i_1 = \frac{V_a - V_o}{10}\]

\[ V_a = 30V\text{ and }V_o = X\]

\[So, i_1 = \frac{30 - X}{10}\]

Similarly,

\[ i_2 = \frac{V_o - V_b}{20}\]

\[ = \frac{X - 12}{20}\]

And

\[ i_3 = \frac{V_o - V_c}{30}\]

\[ = \frac{X - 2}{30}\]

Also, from kirchoff's junction law we have:-

 i₁ = i₂ + i₃

\[\Rightarrow \frac{30 - X}{10} = \frac{X - 12}{20} + \frac{X - 2}{30}\]

\[ \Rightarrow 30 - X = \frac{X - 12}{2} + \frac{X - 2}{3}\]

\[ \Rightarrow 30 - X = \frac{3X - 36 + 2X - 4}{6}\]

\[ \Rightarrow 180 - 6X = 5X - 40\]

\[ \Rightarrow 11X = 220\]

\[ \Rightarrow X = \frac{220}{11} = 20 V\]

Thus, the currents through the three resistors are:-

\[i_1 = \frac{30 - 20}{10} = 1A\]

\[ i_2 = \frac{20 - 12}{20} = \frac{8}{20} = 0 . 4A\]

\[ i_3 = \frac{20 - 2}{30} = \frac{18}{30} = 0 . 6 A\]