Advertisements
Advertisements
प्रश्न
The perimeter of a triangular field is 420 m and its sides are in the ratio 6 : 7 : 8. Find the area of the triangular field.
उत्तर
Given: The perimeter of a triangular field is 420 m and its sides are in the ratio 6 : 7 : 8.
According to the question, Let the sides in meters are a = 6x, b = 7x and c = 8x.
So, perimeter of the triangle = 6x + 7x + 8x
420 = 21x
x = `420/21`
x = 20
Since, the sides of the triangular field are a = 6 × 20 cm = 120 m, b = 7 × 20 m = 140 m and c = 8 × 20 m = 160 m.
Now, semi-perimeter(s) of triangle will be:
`s = 1/2 xx 420 m`
= 210 m
Area of the triangle field = `sqrt(s(s - a)(s - b)(s - c))` ...[Using Heron’s formula]
= `sqrt(210(210 - 120)(210 - 140)(210 - 160))`
= `sqrt(210 xx 90 xx 70 xx 50)`
= `100sqrt(7 xx 3 xx 3^2 xx 7 xx 5)`
= `100 xx 7 xx 3 xx sqrt(15)`
= `2100sqrt(15)`
Therefore, the area of the triangular field is `2100sqrt(15)`.
APPEARS IN
संबंधित प्रश्न
The adjacent sides of a parallelogram ABCD measure 34 cm and 20 cm, and the diagonal AC measures 42 cm. Find the area of the parallelogram.
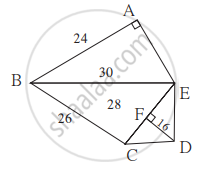
Find the areas of the given plot. (All measures are in metres.)
Find the area of the triangle formed by the points
(1, – 1), (– 4, 6) and (– 3, – 5)
Find the area of the triangle formed by the points
(–10, –4), (–8, –1) and (–3, –5)
If the points A(– 3, 9), B(a, b) and C(4, – 5) are collinear and if a + b = 1, then find a and b
The area of triangle formed by the points (− 5, 0), (0, – 5) and (5, 0) is
Using Heron’s formula, find the area of a triangle whose sides are 1.8 m, 8 m, 8.2 m
Find the area of an equilateral triangle whose perimeter is 180 cm
An advertisement board is in the form of an isosceles triangle with perimeter 36 m and each of the equal sides are 13 m. Find the cost of painting it at ₹ 17.50 per square metre.
The semi-perimeter of a triangle having sides 15 cm, 20 cm and 25 cm is
