Advertisements
Advertisements
प्रश्न
The points A(−5, 4), B(−1, −2) and C(5, 2) are the vertices of an isosceles right-angled triangle where the right angle is at B. Find the coordinates of D so that ABCD is a square
उत्तर
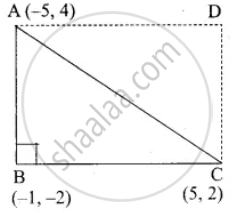
Since ABCD is a square
Mid-point of AC = mid-point of BD
Let the point D be (a, b)
Mid−point of a line = `((x_1 + x_2)/2, (y_1 + y_2)/2)`
Mid−point of AC = `((5 - 5)/2, 2 + 4/2)`
`(0/2, 6/2)` = (0, 3)
Mid−point of BD = `((-1 + "a")/2, (-2 + "b")/2)`
But mid-point of BD = Mid-point of AC
`((-1 + "a")/2, (-2 + "b")/2)` = (0, 3)
`(-1 + "a")/2` = 0
−1 + a = 0
a = 1
`((-2 + b)/2)`
−2 + b = 6
b = 6 + 2 = 8
∴ The vertices D is (1, 8).
APPEARS IN
संबंधित प्रश्न
In the following example find the co-ordinate of point A which divides segment PQ in the ratio a : b.
P(–2, –5), Q(4, 3), a : b = 3 : 4
Point P is the centre of the circle and AB is a diameter . Find the coordinates of point B if coordinates of point A and P are (2, –3) and (–2, 0) respectively.
Find the coordinates of the midpoint of the line segment joining P(0, 6) and Q(12, 20).
Complete the table below the graph with the help of the following graph.

| Sr. No. | First point | Second point | Co-ordinates of first point (x1 , y1) | Co-ordinates of second point (x2 , y2) | `(y_2 - y_2)/(x_2 - x_2)` |
| 1 | C | E | (1, 0) | (3,4) | `4/2=2` |
| 2 | A | B | (-1,-4) | (0,-2) | `2/1 = 2` |
| 3 | B | D | (0,-2) | (2,2) | `4/2=2` |
Two vertices of a triangle are (1, 4) and (3, 1). If the centroid of the triangle is the origin, find the third vertex.
The coordinates of the centroid I of triangle PQR are (2, 5). If Q = (-6, 5) and R = (7, 8). Calculate the coordinates of vertex P.
A lies on the x - axis amd B lies on the y -axis . The midpoint of the line segment AB is (4 , -3). Find the coordinates of A and B .
Find coordinates of midpoint of segment joining (– 2, 6) and (8, 2)
Find the co-ordinates of centroid of a triangle if points D(–7, 6), E(8, 5) and F(2, –2) are the mid-points of the sides of that triangle.
Find the coordinates of the mid-point of the line segment with points A(– 2, 4) and B(–6, –6) on both ends.
