Advertisements
Advertisements
प्रश्न
The radii of two cylinders are in the ratio 2 : 3 and their heights are in the ratio 5 : 3. Calculate the ratio of their curved surface areas.
उत्तर
\[\text{ Let the radii of two cylinders be 2r and 3r, respectively, and their heights be 5h and 3h, respectively .} \]
\[ \text{ Let S_1 and S_2 be the curved surface areas of the two cylinder } . \]
\[ \text{ S_1 = Curved surface area of the cylinder of height 5h and radius 2r } \]
\[ \text{ S_2 = Curved surface area of the cylinder of height 3h and radius 3r} \]
\[ \therefore S_1 : S_2 = 2 \times \pi \times r \times h : 2 \times \pi \times r \times h\]
\[ = \frac{2 \times \pi \times 2r \times 5h}{2 \times \pi \times 3r \times 3h} \]
\[ = 10 : 9\]
APPEARS IN
संबंधित प्रश्न
The students of a Vidyalaya were asked to participate in a competition for making and decorating penholders in the shape of a cylinder with a base, using cardboard. Each penholder was to be of radius 3 cm and height 10.5 cm. The Vidyalaya was to supply the competitors with cardboard. If there were 35 competitors, how much cardboard was required to be bought for the competition?
`["Assume "pi=22/7]`
A rectangular sheet of paper, 44 cm × 20 cm, is rolled along its length to form a cylinder. Find the total surface area of the cylinder thus generated.
The total surface area of a hollow cylinder which is open from both sides is 4620 sq. cm, area of base ring is 115.5 sq. cm and height 7 cm. Find the thickness of the cylinder.
The ratio between the curved surface area and the total surface area of a right circular cylinder is 1 : 2. Find the volume of the cylinder, if its total surface area is 616 cm2.
2.2 cubic dm of brass is to be drawn into a cylindrical wire 0.25 cm in diameter. Find the length of the wire.
The radii of two cylinders are in the ratio 2 : 3 and their heights are in the ratio 5 : 3. Calculate the ratio of their volumes and the ratio of their curved surfaces.
Curved surface area of a cylinder is 1980 cm2 and radius of its base is 15 cm. Find the height of the cylinder. (π = `22/7`)
Find the area of the sheet required to make a cylindrical container which is open at one side and whose diameter is 28 cm and height is 20 cm. Find the approximate area of the sheet required to make a lid of height 2 cm for this container.
Find the lateral surface area, total surface area and the volume of the following cylinders: Radius = 4.2cm, Height = 12cm
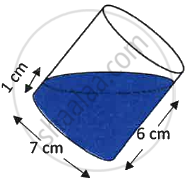
In the adjoining diagram, a tilted right circular cylindrical vessel with base diameter 7 cm contains a liquid. When placed vertically, the height of the liquid in the vessel is the mean of two heights shown in the diagram. Find the area of wet surface, when the cylinder is placed vertically on a horizontal surface. (Use π = `22/7`).