Advertisements
Advertisements
प्रश्न
Three rays (1, 2, 3) of different colours fall normally on one of the sides of an isosceles right angled prism as shown. The refractive index of prism for these rays is 1.39, 1.47 and 1.52 respectively. Find which of these rays get internally reflected and which get only refracted from AC. Trace the paths of rays. Justify your answer with the help of necessary calculations.
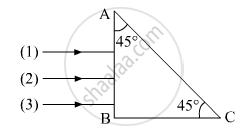
उत्तर
Critical angle for ray 1:
\[\sin\left( c_1 \right) = \frac{1}{\mu_1} = \frac{1}{1 . 39}\]
\[ \Rightarrow c_1 = \sin^{- 1} \left( \frac{1}{1 . 39} \right) = 46 . 00°\]
Similarly, critical angle of ray 2:
\[\sin\left( c_2 \right) = \frac{1}{\mu_2} = \frac{1}{1 . 47}\]
\[ \Rightarrow c_2 = \sin^{- 1} \left( \frac{1}{1 . 47} \right) = 42 . 86°\]
Similarly, critical angle of ray 3:
\[\sin\left( c_3 \right) = \frac{1}{\mu_3} = \frac{1}{1 . 52}\]
\[ \Rightarrow c_3 = \sin^{- 1} \left( \frac{1}{1 . 52} \right) = 41 . 13°\]
The ray 1 will get totally internally reflected from the side AC with critical equal to 46°. Critical angle of ray 1 is greater than that of i (45°) . Critical angle of ray 2 and 3 is less than that of i. Hence, they will be refracted from the side AC.
APPEARS IN
संबंधित प्रश्न
At what angle should a ray of light be incident on the face of a prism of refracting angle 60° so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524.
A ray of light passing from air through an equilateral glass prism undergoes minimum deviation when the angle of incidence is 3/4 th of the angle of prism. Calculate the speed of light in the prism.
A ray of light, incident on an equilateral prism `(μ_g = sqrt3)`moves parallel to the base line of the prism inside it. Find the angle of incidence for this ray.
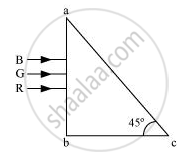
Three light rays red (R), green (G) and blue (B) are incident on a right angled prism ‘abc’ at face ‘ab’. The refractive indices of the material of the prism for red, green and blue wavelengths are 1.39, 1.44 and 1.47 respectively. Out of the three which colour ray will emerge out of face ‘ac’? Justify your answer. Trace the path of these rays after passing through face ‘ab’.
If a piece of paper is placed at the position of a virtual image of a strong light source, will the paper burn after sufficient time? What happens if the image is real? What happens if the image is real but the source is virtual?
A flint glass prism and a crown glass prism are to be combined in such a way that the deviation of the mean ray is zero. The refractive index of flint and crown glasses for the mean ray are 1.620 and 1.518 respectively. If the refracting angle of the flint prism is 6.0°, what would be the refracting angle of the crown prism?
Answer the following question.
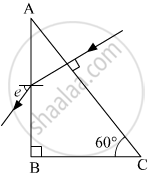
Calculate the angle of emergence (e) of the ray of light incident normally on the face AC of a glass prism ABC of refractive index `sqrt(3)`. How will the angle of emergence change qualitatively, if the ray of light emerges from the prism into a liquid of refractive index 1.3 instead of air?
A prism is made of glass of unknown refractive index. A parallel beam of light is incident on the face of the prism. The angle of minimum deviation is measured to be 40°. What is the refractive index of the material of the prism? The refracting angle of the prism is 60°. If the prism is placed in water (refractive index 1.33), predict the new angle of minimum deviation of a parallel beam of light.
Two prisms ABC and DBC are arranged as shown in the figure.

The critical angles for the two prisms with respect to air are 41.1° and 45° respectively. Trace the path of the ray through the combination.
The maximum value of the index of refraction of a material of a prism which allows the passage of light through it when the refracting angle of the prism is A is ______.
